Advertisements
Advertisements
प्रश्न
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
उत्तर
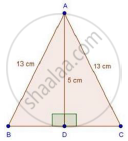
In ΔADB, by Pythagoras theorem
AD2 + BD2 = 132
⇒ 25 + BD2 = 169
⇒ BD2 = 169 − 2 = 144
⇒ BD = `sqrt144` = 12 cm
In ΔADB and ΔADC
∠ADB = ∠ADC [Each 90°]
AB = AC [Each 13 cm]
AD = AD [Common]
Then, ΔADB ≅ ΔADC [By RHS condition]
∴ BD = CD = 12 cm [By c.p.c.t]
Hence, BC = 12 + 12 = 24 cm
APPEARS IN
संबंधित प्रश्न
In an isosceles triangle ABC, AB = AC = 25 cm, BC = 14 cm. Calculate the altitude from A on BC.
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
In an acute-angled triangle, express a median in terms of its sides.
∆ABD is a right triangle right-angled at A and AC ⊥ BD. Show that
(i) AB2 = BC x BD
(ii) AC2 = BC x DC
(iii) AD2 = BD x CD
(iv) `"AB"^2/"AC"^2="BD"/"DC"`
Find the length of the altitude of an equilateral triangle of side 2a cm.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
The co-ordinates of the points A, B and C are (6, 3), (−3, 5) and (4, −2) respectively. P(x, y) is any point in the plane. Show that \[\frac{ar\left( ∆ PBC \right)}{ar\left( ∆ ABC \right)} = \left| \frac{x + y - 2}{7} \right|\]
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

