Advertisements
Advertisements
प्रश्न
Find the altitude of an equilateral triangle of side 8 cm.
उत्तर
Let ABC be an equilateral triangle of side 8 cm
AB = BC = CA = 8 cm ...(All sides of an equilateral triangle is equal)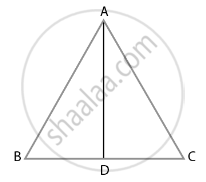
Draw altitude AD which is perpendicular to BC.
Then, D is the mid-point of BC.
∴ BD = CD = `1/2`
BC = `8/2` = 4 cm
Now, by Pythagoras theorem
AB2 = AD2 + BD2
⇒ (8)2 = AD2 + (4)2
⇒ 64 = AD2 + 16
⇒ AD = 64 – 16 = 48
⇒ AD = `sqrt(48)` = `4sqrt(3)` cm.
Hence, altitude of an equilateral triangle is `4sqrt(3)` cm.
APPEARS IN
संबंधित प्रश्न
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
Using Pythagoras theorem determine the length of AD in terms of b and c shown in Figure.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
In right-angled triangle ABC in which ∠C = 90°, if D is the mid-point of BC, prove that AB2 = 4AD2 − 3AC2.
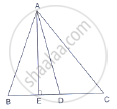
In Figure, D is the mid-point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED
= x, AD = p and AE = h, prove that:
(i) `b^2 = p^2 + ax + a^2/4`
(ii) `c^2 = p^2 - ax + a^2/4`
(iii) `b^2 + c^2 = 2p^2 + a^2/2`
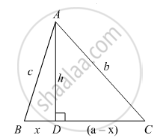
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
An aeroplane leaves an airport and flies due north at a speed of 1000km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1 hours?
In an equilateral triangle with side a, prove that area = `sqrt3/4` 𝑎2
A man goes 12m due south and then 35m due west. How far is he from the starting point.
