Advertisements
Advertisements
प्रश्न
In figure, if AB || DC and AC and PQ intersect each other at the point O, prove that OA . CQ = OC . AP
उत्तर
According to the question,
AC and PQ intersect each other at the point O and AB || DC.
From ∆AOP and ∆COQ,
∠AOP = ∠COQ ...[Since they are vertically opposite angles]
∠APO = ∠CQO ...[Since, AB || DC and PQ is transversal, the angles are alternate angles]
∴ ∆AOP ∼ ∆COQ ...[Using AAA similarity criterion]
Then, since, corresponding sides are proportional
We have,
`("OA")/("OC") = ("AP")/("CQ")`
OA × CQ = OC × AP
Hence proved.
APPEARS IN
संबंधित प्रश्न
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
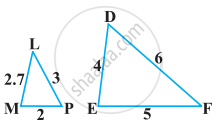
In the following figure, ΔODC ∼ ΔOBA, ∠BOC = 125° and ∠CDO = 70°. Find ∠DOC, ∠DCO and ∠OAB.

S and T are point on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ∼ ΔRTS.
In the following Figure, DE || BC such that AE = (1/4) AC. If AB = 6 cm, find AD.
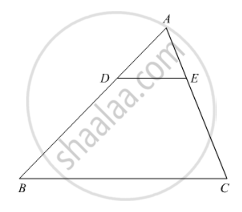
D is the mid-point of side BC of a ΔABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3 : 1
The sides of certain triangles are given below. Determine which of them right triangles are.
1.4cm, 4.8cm, 5cm
State the AAA-similarity criterion
In a ∆PQR, PR2 – PQ2 = QR2 and M is a point on side PR such that QM ⊥ PR. Prove that QM2 = PM × MR.
In figure, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to ______.
If in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle, can you say that the two triangles will be similar? Why?
