Advertisements
Advertisements
प्रश्न
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
उत्तर
AB = BC ......[Given]
∴ ∠A = ∠C ......[Isosceles triangle theorem]
Let ∠A = ∠C = x ......(i)
In ∆ABC, ∠A + ∠B + ∠C = 180° ......[Sum of the measures of the angles of a triangle is 180°]
∴ x + 90° + x = 180° ......[From (i)]
∴ 2x = 90°
∴ x = `90^circ/2` ......[From (i)]
∴ x = 45°
∴ ∠BAC = ∠BCA = 45°
∴ ∆ABC is a 45° – 45° – 90° triangle.
∴ AB = BC = `1/sqrt(2) xx "AC"` ......[Side opposite to 45°]
= `1/sqrt(2) xx 2sqrt(2)`
∴ `l("AB")` = 2 units
APPEARS IN
संबंधित प्रश्न
The sides of triangle is given below. Determine it is right triangle or not.
a = 1.6 cm, b = 3.8 cm and c = 4 cm
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
In a ΔABC, AB = BC = CA = 2a and AD ⊥ BC. Prove that
(i) AD = a`sqrt3`
(ii) Area (ΔABC) = `sqrt3` a2
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
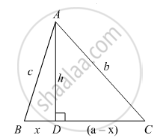
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
In a right ∆ABC right-angled at C, if D is the mid-point of BC, prove that BC2 = 4(AD2 − AC2).
In a quadrilateral ABCD, ∠B = 90°, AD2 = AB2 + BC2 + CD2, prove that ∠ACD = 90°.
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
State Pythagoras theorem
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
In an equilateral triangle with side a, prove that area = `sqrt3/4` 𝑎2
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
Find the side and perimeter of a square whose diagonal is `13sqrt2` cm.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?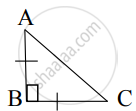
A girl walks 200m towards East and then 150m towards North. The distance of the girl from the starting point is ______.
In a ΔABC, ∠CAB is an obtuse angle. P is the circumcentre of ∆ABC. Prove that ∠CAB – ∠PBC = 90°.
