Advertisements
Advertisements
प्रश्न
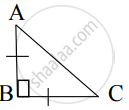
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?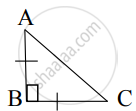
उत्तर
AB = BC ......[Given]
∴ ∠A = ∠C ......[Isosceles triangle theorem]
Let ∠A = ∠C = x
In ∆ABC,
∠A + ∠B + ∠C = 180° ...[Sum of the measures of the angles of a triangle is 180°]
∴ x + 90° + x = 180°
∴ 2x = 90°
∴ x = `(90°)/2`
∴ x = 45°
∴ m∠A = 45°
संबंधित प्रश्न
The sides of triangle is given below. Determine it is right triangle or not.
a = 8 cm, b = 10 cm and c = 6 cm
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
ABCD is a square. F is the mid-point of AB. BE is one third of BC. If the area of ΔFBE = 108 cm2, find the length of AC.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
In an acute-angled triangle, express a median in terms of its sides.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
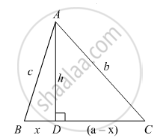
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
An aeroplane leaves an airport and flies due north at a speed of 1000km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1 hours?
State the converse of Pythagoras theorem.
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
A man goes 12m due south and then 35m due west. How far is he from the starting point.
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
The co-ordinates of the points A, B and C are (6, 3), (−3, 5) and (4, −2) respectively. P(x, y) is any point in the plane. Show that \[\frac{ar\left( ∆ PBC \right)}{ar\left( ∆ ABC \right)} = \left| \frac{x + y - 2}{7} \right|\]
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
Find the side and perimeter of a square whose diagonal is `13sqrt2` cm.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `5sqrt(2)` , then what is the height of ∆ABC?