Advertisements
Advertisements
प्रश्न
In a right ∆ABC right-angled at C, if D is the mid-point of BC, prove that BC2 = 4(AD2 − AC2).
उत्तर
It is given that ∆ABC is a right-angled at C and D is the mid-point of BC.

In the right angled triangle ADC, we will use Pythagoras theorem,
AD2 = DC2 + AC2 ..........(1)
Since D is the midpoint of BC, we have
`"DC"/="BC"/2`
Substituting `"DC"/="BC"/2` in equation (1), we get
`"AD"^2=("BC"/2)^2+"AC"^2`
`"AD"^2="BC"^2/4+"AC"^2`
4AD2 = BC2 + 4AC2
BC2 = 4AD2 - 4AC2
BC2 = 4(AD2 - AC2)
APPEARS IN
संबंधित प्रश्न
The sides of triangle is given below. Determine it is right triangle or not.
a = 7 cm, b = 24 cm and c = 25 cm
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
Determine whether the triangle having sides (a − 1) cm, 2`sqrta` cm and (a + 1) cm is a right-angled
triangle.
Find the length of the altitude of an equilateral triangle of side 2a cm.
A man goes 12m due south and then 35m due west. How far is he from the starting point.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?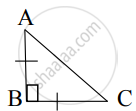
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `5sqrt(2)` , then what is the height of ∆ABC?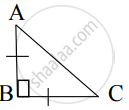
Find the height of an equilateral triangle having side 4 cm?
