Advertisements
Advertisements
Question
In a right ∆ABC right-angled at C, if D is the mid-point of BC, prove that BC2 = 4(AD2 − AC2).
Solution
It is given that ∆ABC is a right-angled at C and D is the mid-point of BC.

In the right angled triangle ADC, we will use Pythagoras theorem,
AD2 = DC2 + AC2 ..........(1)
Since D is the midpoint of BC, we have
`"DC"/="BC"/2`
Substituting `"DC"/="BC"/2` in equation (1), we get
`"AD"^2=("BC"/2)^2+"AC"^2`
`"AD"^2="BC"^2/4+"AC"^2`
4AD2 = BC2 + 4AC2
BC2 = 4AD2 - 4AC2
BC2 = 4(AD2 - AC2)
APPEARS IN
RELATED QUESTIONS
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
The sides of triangle is given below. Determine it is right triangle or not.
a = 9 cm, b = l6 cm and c = 18 cm
The sides of triangle is given below. Determine it is right triangle or not.
a = 8 cm, b = 10 cm and c = 6 cm
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
In right-angled triangle ABC in which ∠C = 90°, if D is the mid-point of BC, prove that AB2 = 4AD2 − 3AC2.
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
Find the length of the altitude of an equilateral triangle of side 2a cm.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?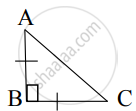
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
In a ΔABC, ∠CAB is an obtuse angle. P is the circumcentre of ∆ABC. Prove that ∠CAB – ∠PBC = 90°.
