Question

In ∆ABC, ∠A is obtuse, PB ⊥ AC and QC ⊥ AB. Prove that:

(i) AB ✕ AQ = AC ✕ AP

(ii) BC² = (AC ✕ CP + AB ✕ BQ)

Answer 1

Given: ΔABC where ∠BAC is obtuse. PB ⊥AC and QC⊥AB.

To prove:

(i) AB × AQ = AC × AP and

(ii) BC² = AC × CP + AB × BQ

Proof: In ΔACQ and ΔABP,

∠CAQ = ∠BAP (Vertically opposite angles)

∠Q = ∠P (= 90°)

∴ ΔACQ ∼ ΔABP [AA similarity test]

`rArr"CQ"/"BP"="AC"/"AB"="AQ"/"AP"`            [Corresponding sides are in the same proportion]

`"AC"/"AB"="AQ"/"AP"`

⇒ AQ x AB = AC x AP              .....(1)

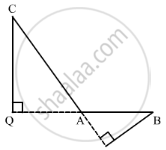
In right ΔBCQ,

⇒ BC² = CQ² + QB²

⇒ BC² = CQ² + (QA + AB)²

⇒ BC² = CQ² + QA² + AB² + 2QA × AB

⇒ BC² = AC² + AB² + QA × AB + QA × AB              [In right ΔACQ, CQ² + QA² = AC²]

⇒ BC² = AC² + AB² + QA × AB + AC × AP         (Using (1))

⇒ BC² = AC (AC + AP) + AB (AB + QA)

⇒ BC² = AC × CP + AB × BQ