Advertisements
Advertisements
Question
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
Solution
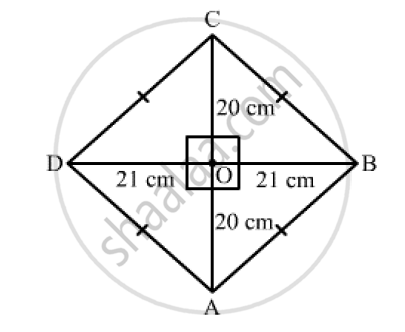
Suppose ABCD is a rhombus.
We know that the diagonals of a rhombus perpendicularly bisect each other.
∴ ∠๐ด๐๐ต = 90°, ๐ด๐ = 20 ๐๐ ๐๐๐ ๐ต๐ = 21 ๐๐
Now, In right triangle AOB
By using Pythagoras theorem we have
`AB^2=AQ^2+OB^2`
=`20^2+21^2`
=`400+441`
=`841`
∴ `AB^2=841`
⇒ `AB=29 cm`
Since, all the sides of a rhombus are equal.
Hence, AB = BC = CD = DA = 29 cm
APPEARS IN
RELATED QUESTIONS
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
In a ΔABC, AB = BC = CA = 2a and AD ⊥ BC. Prove that
(i) AD = a`sqrt3`
(ii) Area (ΔABC) = `sqrt3` a2
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
State the converse of Pythagoras theorem.
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
A man goes 12m due south and then 35m due west. How far is he from the starting point.
From given figure, In โABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
Find the altitude of an equilateral triangle of side 8 cm.
