Advertisements
Advertisements
Question
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
Solution
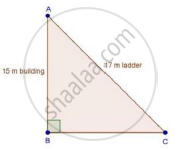
In ΔABC, by Pythagoras theorem
AB2 + BC2 = AC2
⇒ 152 + BC2 = 172
⇒ 225 + BC2 = 289
⇒ BC2 = 289 − 225
⇒ BC2 = 64
⇒ BC = 8 m
∴ Distance of the foot of the ladder from building = 8 m
APPEARS IN
RELATED QUESTIONS
The sides of triangle is given below. Determine it is right triangle or not.
a = 8 cm, b = 10 cm and c = 6 cm
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
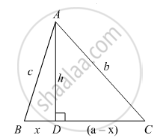
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
In a right ∆ABC right-angled at C, if D is the mid-point of BC, prove that BC2 = 4(AD2 − AC2).
Determine whether the triangle having sides (a − 1) cm, 2`sqrta` cm and (a + 1) cm is a right-angled
triangle.
State the converse of Pythagoras theorem.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
