Advertisements
Advertisements
Question
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
Solution
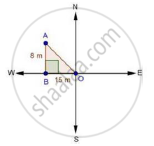
Let the starting point of the man be O and final point be A.
∴ In ΔABO, by Pythagoras theorem AO2 = AB2 + BO2
⇒ AO2 = 82 + 152
⇒ AO2 = 64 + 225 = 289
⇒ AO = `sqrt289` = 17m
∴ He is 17m far from the starting point.
APPEARS IN
RELATED QUESTIONS
In an isosceles triangle ABC, AB = AC = 25 cm, BC = 14 cm. Calculate the altitude from A on BC.
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
∆ABD is a right triangle right-angled at A and AC ⊥ BD. Show that
(i) AB2 = BC x BD
(ii) AC2 = BC x DC
(iii) AD2 = BD x CD
(iv) `"AB"^2/"AC"^2="BD"/"DC"`
State Pythagoras theorem
Find the length of the altitude of an equilateral triangle of side 2a cm.
A man goes 12m due south and then 35m due west. How far is he from the starting point.
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
Find the side and perimeter of a square whose diagonal is `13sqrt2` cm.
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

