Advertisements
Advertisements
Question
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

Options
60°
45°
30°
15°
Solution
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is 60°.

Explanation:
cos P = `(PQ)/(PR)`
= `4/8`
= `1/2`
cos P = cos 60°
`\implies` P = 60°
APPEARS IN
RELATED QUESTIONS
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
The sides of triangle is given below. Determine it is right triangle or not.
a = 7 cm, b = 24 cm and c = 25 cm
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
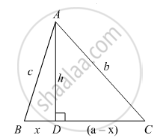
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `2sqrt(2)` then l (AB) = ?
Find the altitude of an equilateral triangle of side 8 cm.
