Advertisements
Advertisements
Question
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Solution
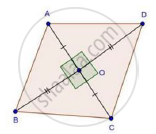
We have,
ABCD is a rhombus with diagonals AC = 10 cm and BD = 24 cm
We know that diagonal of a rhombus bisect each other at 90°
∴ AO = OC = 5 cm and BO = OD = 12 cm
In ΔAOB, by Pythagoras theorem
AB2 = AO2 + BO2
⇒ AB2 = 52 + 122
⇒ AB2 = 25 + 144 = 169
⇒ AB = `sqrt169` = 13 cm
APPEARS IN
RELATED QUESTIONS
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
In ∆ABC, ∠A is obtuse, PB ⊥ AC and QC ⊥ AB. Prove that:
(i) AB ✕ AQ = AC ✕ AP
(ii) BC2 = (AC ✕ CP + AB ✕ BQ)
An aeroplane leaves an airport and flies due north at a speed of 1000km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1 hours?
Find the length of the altitude of an equilateral triangle of side 2a cm.
In an equilateral triangle with side a, prove that area = `sqrt3/4` 𝑎2
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
Find the height of an equilateral triangle having side 4 cm?
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

