Advertisements
Advertisements
Question
Find the height of an equilateral triangle having side 4 cm?
Solution
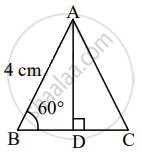
Let ∆ABC be the given equilateral triangle.
∴ ∠B = 60° ......[Angle of an equilateral triangle]
Let AD ⊥ BC, B – D – C.
In ∆ABD, ∠B = 60°, ∠ADB = 90°
∴ ∠BAD = 30° ......[Remaining angle of a triangle]
∴ ∆ABD is a 30° – 60° – 90° triangle.
∴ AD = `sqrt(3)/2` AB ......[Side opposite to 60°]
= `sqrt(3)/2 xx 4`
= `2sqrt(3)` units
∴ The height of the equilateral triangle is `2sqrt(3)` units.
APPEARS IN
RELATED QUESTIONS
The sides of triangle is given below. Determine it is right triangle or not.
a = 8 cm, b = 10 cm and c = 6 cm
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
In an acute-angled triangle, express a median in terms of its sides.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
In right-angled triangle ABC in which ∠C = 90°, if D is the mid-point of BC, prove that AB2 = 4AD2 − 3AC2.
Determine whether the triangle having sides (a − 1) cm, 2`sqrta` cm and (a + 1) cm is a right-angled
triangle.
State the converse of Pythagoras theorem.
If D, E, F are the respectively the midpoints of sides BC, CA and AB of ΔABC. Find the ratio of the areas of ΔDEF and ΔABC.
Find the length of the altitude of an equilateral triangle of side 2a cm.
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
The co-ordinates of the points A, B and C are (6, 3), (−3, 5) and (4, −2) respectively. P(x, y) is any point in the plane. Show that \[\frac{ar\left( ∆ PBC \right)}{ar\left( ∆ ABC \right)} = \left| \frac{x + y - 2}{7} \right|\]
A girl walks 200m towards East and then 150m towards North. The distance of the girl from the starting point is ______.
Find the altitude of an equilateral triangle of side 8 cm.
