Advertisements
Advertisements
рдкреНрд░рд╢реНрди
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
рдЙрддреНрддрд░
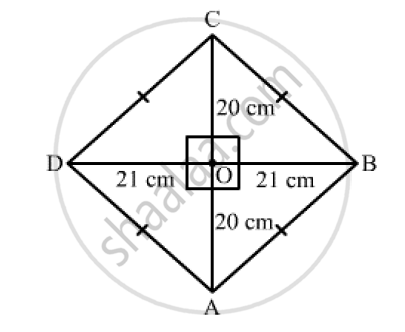
Suppose ABCD is a rhombus.
We know that the diagonals of a rhombus perpendicularly bisect each other.
∴ ∠ЁЭР┤ЁЭСВЁЭР╡ = 90°, ЁЭР┤ЁЭСВ = 20 ЁЭСРЁЭСЪ ЁЭСОЁЭСЫЁЭСС ЁЭР╡ЁЭСВ = 21 ЁЭСРЁЭСЪ
Now, In right triangle AOB
By using Pythagoras theorem we have
`AB^2=AQ^2+OB^2`
=`20^2+21^2`
=`400+441`
=`841`
∴ `AB^2=841`
⇒ `AB=29 cm`
Since, all the sides of a rhombus are equal.
Hence, AB = BC = CD = DA = 29 cm
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНрди
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
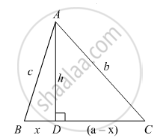
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
In тИЖABC, ∠A is obtuse, PB ⊥ AC and QC ⊥ AB. Prove that:
(i) AB тЬХ AQ = AC тЬХ AP
(ii) BC2 = (AC тЬХ CP + AB тЬХ BQ)
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
An aeroplane leaves an airport and flies due north at a speed of 1000km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1 hours?
Determine whether the triangle having sides (a − 1) cm, 2`sqrta` cm and (a + 1) cm is a right-angled
triangle.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
In an equilateral triangle with side a, prove that area = `sqrt3/4` ЁЭСО2
In a ΔABC, ∠CAB is an obtuse angle. P is the circumcentre of тИЖABC. Prove that ∠CAB – ∠PBC = 90°.
