Advertisements
Advertisements
प्रश्न
In an equilateral triangle with side a, prove that area = `sqrt3/4` 𝑎2
उत्तर
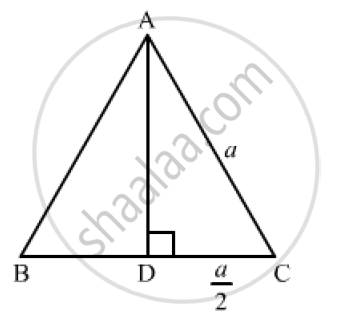
We know that the altitude of an equilateral triangle bisects the side on which it stands and forms right angled triangles with the remaining sides.
Suppose ABC is an equilateral triangle having AB =BC = CA = a.
Suppose AD is the altitude drawn from the vertex A to the side BC.
So, It will bisects the side BC
∴` DC=1/2 a `
Now, In right triangle ADC
By using Pythagoras theorem, we have
`AC^2=CD^2+DA^2`
⇒` a^2-(1/2 a)^2+DA^2`
⇒ `DA^2=a^2-1/4 a^2`
⇒` DA^2=3/4 a^2`
⇒`DA=sqrt3/2 a`
𝑁𝑜𝑤,𝑎𝑟𝑒𝑎 (Δ𝐴𝐵𝐶)=`1/2xxBCxxAD`
=` 1/2xxaxxsqrt3/2 a`
=`sqrt3/4 a^2`
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
Using Pythagoras theorem determine the length of AD in terms of b and c shown in Figure.
In a quadrilateral ABCD, ∠B = 90°, AD2 = AB2 + BC2 + CD2, prove that ∠ACD = 90°.
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
Find the side and perimeter of a square whose diagonal is `13sqrt2` cm.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `5sqrt(2)` , then what is the height of ∆ABC?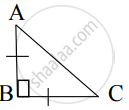
Find the altitude of an equilateral triangle of side 8 cm.
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

