Advertisements
Advertisements
प्रश्न
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
उत्तर
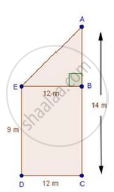
We have,
AC = 14 m, DC = 12m and ED = BC = 9m
Construction: Draw EB ⊥ AC
∴ AB = AC – BC = 14 – 9 = 5m
And, EB = DC = 12 m
In ΔABE, by Pythagoras theorem,
AE2 = AB2 + BE2
⇒ AE2 = 52 + 122
⇒ AE2 = 25 + 144 = 169
⇒ AE = `sqrt169` = 13 m
∴ Distance between their tops = 13 m
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC with sides BC = 7 cm, ∠B = 45° and ∠A = 105°. Then construct a triangle whose sides are `3/4` times the corresponding sides of ∆ABC.
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
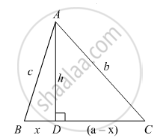
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
In a quadrilateral ABCD, ∠B = 90°, AD2 = AB2 + BC2 + CD2, prove that ∠ACD = 90°.
ΔABC~ΔDEF such that ar(ΔABC) = 64 cm2 and ar(ΔDEF) = `169cm^2`. If BC = 4cm, find EF.
Find the length of each side of a rhombus are 40 cm and 42 cm. find the length of each side of the rhombus.
From given figure, In ∆ABC, AB ⊥ BC, AB = BC then m∠A = ?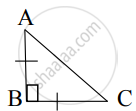
In the given figure, ΔPQR is a right triangle right angled at Q. If PQ = 4 cm and PR = 8 cm, then P is ______.

