Advertisements
Online Mock Tests
Chapters
2: Polynomials
3: Pair of Linear Equations in Two Variables
4: Quadratic Equations
5: Arithmetic Progression
6: Co-Ordinate Geometry
▶ 7: Triangles
8: Circles
9: Constructions
10: Trigonometric Ratios
11: Trigonometric Identities
12: Trigonometry
13: Areas Related to Circles
14: Surface Areas and Volumes
15: Statistics
16: Probability
![RD Sharma solutions for Mathematics [English] Class 10 chapter 7 - Triangles RD Sharma solutions for Mathematics [English] Class 10 chapter 7 - Triangles - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
Advertisements
Solutions for Chapter 7: Triangles
Below listed, you can find solutions for Chapter 7 of CBSE RD Sharma for Mathematics [English] Class 10.
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.1 [Pages 2 - 3]
All circles are ______.
congruent
similar
All squares are ______.
similar
congruent
All ______ triangles are similar.
isosceles
equilateral
Two triangles are similar, if their corresponding angles are .......... (proportional, equal)
Two triangles are similar, if their corresponding sides are .......... (proportional, equal)
Two polygons of the same number of sides are similar, if (a) their corresponding angles are ______ and (b) their corresponding sides are ______. (equal, proportional)
Write the truth value (T/F) of each of the following statement
Any two similar figures are congruent.
Write the truth value (T/F) of each of the following statement
Any two congruent figures are similar.
Write the truth value (T/F) of each of the following statement
Two polygons are similar, if their corresponding sides are proportional.
Write the truth value (T/F) of each of the following statement
Two polygons are similar if their corresponding angles are proportional.
Write the truth value (T/F) of each of the following statement
Two triangles are similar if their corresponding sides are proportional.
Write the truth value (T/F) of each of the following statement
Two triangles are similar if their corresponding angles are proportional.
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.2 [Pages 19 - 31]
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 6 cm, DB = 9 cm and AE = 8 cm, find AC.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB"=3/4` and AC = 15 cm, find AE
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"DB"=2/3` and AC = 18 cm, find AE
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 4, AE = 8, DB = x – 4, and EC = 3x – 19, find x.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8cm, AB = 12 cm and AE = 12 cm, find CE.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 4 cm, DB = 4.5 cm and AE = 8 cm, find AC.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 2 cm, AB = 6 cm and AC = 9 cm, find AE.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If `"AD"/"BD"=4/5` and EC = 2.5 cm, find AE
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = x, DB = x − 2, AE = x + 2 and EC = x − 1, find the value of x.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x − 7, DB = 5x − 3, AE = 4x − 3 and EC = (3x − 1), find the value of x.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 4x − 3, AE = 8x – 7, BD = 3x – 1 and CE = 5x − 3, find the volume of x.
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 2.5 cm, BD = 3.0 cm and AE = 3.75 cm, find the length of AC.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 2cm, AD = 8cm, AE = 12 cm and AC = l8cm.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 5.6cm, AD = 1.4cm, AC= 7.2 cm and AE = 1.8 cm.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 10.8 cm, BD = 4.5 cm, AC = 4.8 cm and AE = 2.8 cm.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AD = 5.7 cm, BD = 9.5 cm, AE = 3.3 cm and EC = 5.5 cm.
In a ΔABC, P and Q are points on sides AB and AC respectively, such that PQ || BC. If AP = 2.4 cm, AQ = 2 cm, QC = 3 cm and BC = 6 cm, find AB and PQ.
In a ΔABC, D and E are points on AB and AC respectively such that DE || BC. If AD = 2.4cm, AE = 3.2 cm, DE = 2cm and BC = 5 cm, find BD and CE.
In below Fig., state if PQ || EF.
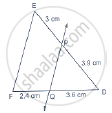
M and N are points on the sides PQ and PR respectively of a ΔPQR. For the following case, state whether MN || QR
PM = 4cm, QM = 4.5 cm, PN = 4 cm and NR = 4.5 cm
In three line segments OA, OB, and OC, points L, M, N respectively are so chosen that LM || AB and MN || BC but neither of L, M, N nor of A, B, C are collinear. Show that LN ||AC.
If D and E are points on sides AB and AC respectively of a ΔABC such that DE || BC and BD = CE. Prove that ΔABC is isosceles.
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.3 [Pages 31 - 32]
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If BD = 2.5cm, AB = 5cm and AC = 4.2cm, find DC.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If BD = 2cm, AB = 5cm and DC = 3cm, find AC.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AB = 3.5 cm, AC = 4.2 cm and DC = 2.8 cm, find BD.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AB = 10 cm, AC =14 cm and BC =6 cm, find BD and DC.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AC = 4.2 cm, DC = 6 cm and 10 cm, find AB
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AB = 5.6 cm, AC = 6cm and DC = 3cm, find BC.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AD = 5.6 cm, BC = 6cm and BD = 3.2 cm, find AC.
In a ΔABC, AD is the bisector of ∠A, meeting side BC at D.
If AB = 10cm, AC = 6 cm and BC = 12 cm, find BD and DC.
In the following Figure, AE is the bisector of the exterior ∠CAD meeting BC produced in E. If AB = 10cm, AC = 6cm and BC = 12 cm, find CE.
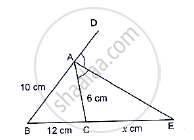
In the following Figure, ΔABC is a triangle such that `"AB"/"AC"="BD"/"DC"`, ∠B = 70°, ∠C = 50°. Find ∠BAD.
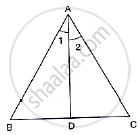
In the following figure, check whether AD is the bisector of ∠A of ∆ABC in the following case:
AB = 5 cm, AC = 10 cm, BD = 1.5 cm and CD = 3.5 cm

In the following figure, check whether AD is the bisector of ∠A of ∆ABC in the following case:
AB = 4 cm, AC = 6 cm, BD = 1.6 cm and CD = 2.4 cm

In the following figure, check whether AD is the bisector of ∠A of ∆ABC in the following case:
AB = 8 cm, AC = 24 cm, BD = 6 cm and BC = 24 cm

In the following figure, check whether AD is the bisector of ∠A of ∆ABC in the following case:
AB = 6 cm, AC = 8 cm, BD = 1.5 cm and CD = 2 cm

In the following figure, check whether AD is the bisector of ∠A of ∆ABC in the following case:
AB = 5 cm, AC = 12 cm, BD = 2.5 cm and BC = 9 cm

In ΔABC , if ∠1 = ∠2, prove that `"AB"/"AC"="BD"/"DC"`
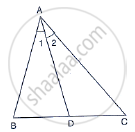
D, E and F are the points on sides BC, CA and AB respectively of ΔABC such that AD bisects ∠A, BE bisects ∠B and CF bisects ∠C. If AB = 5 cm, BC = 8 cm and CA = 4 cm, determine AP, CE and BD.
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.4 [Page 37]
In below figure, If AB || CD, find the value of x.

In the below figure, If AB || CD, find the value of x.

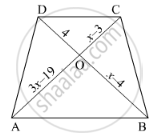
In below figure, AB || CD. If OA = 3x – 19, OB = x – 4, OC = x – 3 and OD = 4, find x.
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.5 [Pages 73 - 76]
In figure, ∆ACB ~ ∆APQ. If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm, AP = 2.8 cm, find CA and AQ.
In the following figure, AB || QR. Find the length of PB.

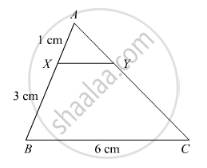
In the following figure, XY || BC. Find the length of XY.
In a right angled triangle with sides a and b and hypotenuse c, the altitude drawn on the hypotenuse is x. Prove that ab = cx.
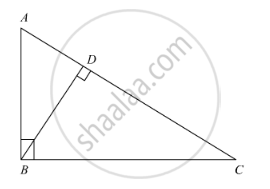
In the following Figure, ∠ABC = 90° and BD ⊥ AC. If BD = 8 cm and AD = 4 cm, find CD.

In the following Figure, ∠ABC = 90° and BD ⊥ AC. If AB = 5.7 cm, BD = 3.8 cm and CD = 5.4 cm, find BC.
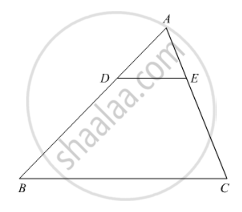
In the following Figure, DE || BC such that AE = (1/4) AC. If AB = 6 cm, find AD.
In the following figure, PA, QB and RC are each perpendicular to AC. Prove that `1/x+1/z=1/y`

Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using similarity criterion for two triangles, show that `"OA"/"OC"="OB"/"OD"`.
In the following figure, ABC and AMP are two right triangles, right-angled at B and M respectively, prove that:

- ΔABC ~ ΔAMP
- `("CA")/("PA") = ("BC")/("MP")`
In below figure, ∠A = ∠CED, Prove that ΔCAB ~ ΔCED. Also, find the value of x.
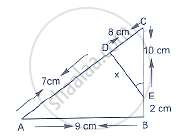
The perimeters of two similar triangles are 25 cm and 15 cm respectively. If one side of first triangle is 9 cm, what is the corresponding side of the other triangle?
In ΔABC and ΔDEF, it is being given that: AB = 5 cm, BC = 4 cm and CA = 4.2 cm; DE=10cm, EF = 8 cm and FD = 8.4 cm. If AL ⊥ BC and DM ⊥ EF, find AL: DM.
D and E are the points on the sides AB and AC respectively of a ΔABC such that: AD = 8 cm, DB = 12 cm, AE = 6 cm and CE = 9 cm. Prove that BC = 5/2 DE.
D is the mid-point of side BC of a ΔABC. AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE : EX = 3 : 1
ABCD is a parallelogram and APQ is a straight line meeting BC at P and DC produced at Q. Prove that the rectangle obtained by BP and DQ is equal to the AB and BC.
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the mid-points of AB, AC, CD and BD respectively, show that PQRS is a rhombus.
In ΔABC, AL and CM are the perpendiculars from the vertices A and C to BC and AB respectively. If AL and CM intersect at O, prove that:
(i) ΔOMA and ΔOLC
(ii) `"OA"/"OC"="OM"/"OL"`
In Figure below, if AB ⊥ BC, DC ⊥ BC and DE ⊥ AC, Prove that Δ CED ~ ABC.
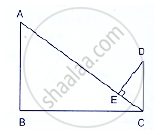
In an isosceles ΔABC, the base AB is produced both the ways to P and Q such that AP × BQ = AC2. Prove that ΔAPC ~ ΔBCQ.
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2m/sec. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.
A vertical stick of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
In below Figure, ΔABC is right angled at C and DE ⊥ AB. Prove that ΔABC ~ ΔADE and Hence find the lengths of AE and DE.

In Fig below we have AB || CD || EF. If AB = 6 cm, CD = x cm, EF = 10 cm, BD = 4 cm and DE = y cm, calculate the values of x and y.

RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.6 [Pages 94 - 96]
If ∆ABC ~ ∆DEF such that area of ∆ABC is 16cm2 and the area of ∆DEF is 25cm2 and BC = 2.3 cm. Find the length of EF.
If ∆ABC is similar to ∆DEF such that ∆DEF = 64 cm2 , DE = 5.1 cm and area of ∆ABC = 9 cm2 . Determine the area of AB
Triangles ABC and DEF are similar If AC = 19cm and DF = 8 cm, find the ratio of the area of two triangles.
Triangles ABC and DEF are similar If area (ΔABC) = 36 cm2, area (ΔDEF) = 64 cm2 and DE = 6.2 cm, find AB.
Triangles ABC and DEF are similar If AB = 1.2 cm and DE = 1.4 cm, find the ratio of the areas of ΔABC and ΔDEF.
In figure below ΔACB ~ ΔAPQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm,
find CA and AQ. Also, find the area (ΔACB): area (ΔAPQ)

The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
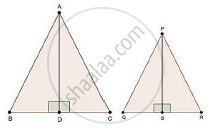
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, find the longest side of the smaller triangle.
The areas of two similar triangles are 25 cm2 and 36 cm2 respectively. If the altitude of the first triangle is 2.4 cm, find the corresponding altitude of the other.
The corresponding altitudes of two similar triangles are 6 cm and 9 cm respectively. Find the ratio of their areas.
ABC is a triangle in which ∠A =90°, AN⊥ BC, BC = 12 cm and AC = 5cm. Find the ratio of the areas of ΔANC and ΔABC.
In Figure, DE || BC If DE = 4 cm, BC = 6 cm and Area (ΔADE) = 16 cm2, find the area of ΔABC.

In Figure, DE || BC If DE = 4cm, BC = 8 cm and Area (ΔADE) = 25 cm2, find the area of ΔABC.
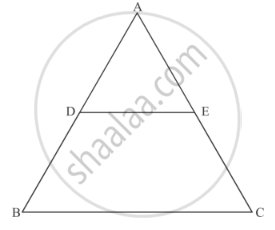
In the given figure, DE || BC and DE : BC = 3 : 5. Calculate the ratio of the areas of ∆ADE and the trapezium BCED

In ΔABC, D and E are the mid-points of AB and AC respectively. Find the ratio of the areas of ΔADE and ΔABC
The areas of two similar triangles are 100 cm2 and 49 cm2 respectively. If the altitude the bigger triangle is 5 cm, find the corresponding altitude of the other.
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.
If ΔABC ~ ΔDEF such that AB = 5 cm, area (ΔABC) = 20 cm2 and area (ΔDEF) = 45 cm2, determine DE.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divides ΔABC into two parts equal in area. Find `(BP)/(AB)`
The areas of two similar triangles ABC and PQR are in the ratio 9:16. If BC = 4.5 cm, find the length of QR.
ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1 cm, PB = 3 cm, AQ = 1.5 cm, QC = 4.5 m, prove that area of ΔAPQ is one- sixteenth of the area of ABC.
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
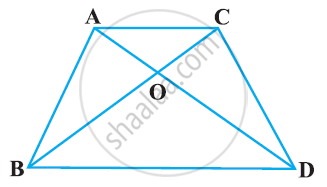
ABCD is a trapezium in which AB || CD. The diagonals AC and BD intersect at O. Prove that: (i) ΔAOB and ΔCOD (ii) If OA = 6 cm, OC = 8 cm,
Find:(a) `("area"(triangleAOB))/("area"(triangleCOD))`
(b) `("area"(triangleAOD))/("area"(triangleCOD))`
If D is a point on the side AB of ΔABC such that AD : DB = 3.2 and E is a Point on BC such that DE || AC. Find the ratio of areas of ΔABC and ΔBDE.
In ABC, P divides the side AB such that AP : PB = 1 : 2. Q is a point in AC such that PQ || BC. Find the ratio of the areas of ΔAPQ and trapezium BPQC.
If ΔABC and ΔBDE are equilateral triangles, where D is the mid-point of BC, find the ratio of areas of ΔABC and ΔBDE.
AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (ΔADE): Area (ΔABC) = 3: 4
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.7 [Pages 119 - 121]
If the sides of a triangle are 3 cm, 4 cm, and 6 cm long, determine whether the triangle is a right-angled triangle.
The sides of triangle is given below. Determine it is right triangle or not.
a = 7 cm, b = 24 cm and c = 25 cm
The sides of triangle is given below. Determine it is right triangle or not.
a = 9 cm, b = l6 cm and c = 18 cm
The sides of triangle is given below. Determine it is right triangle or not.
a = 1.6 cm, b = 3.8 cm and c = 4 cm
The sides of triangle is given below. Determine it is right triangle or not.
a = 8 cm, b = 10 cm and c = 6 cm
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
A ladder 17 m long reaches a window of a building 15 m above the ground. Find the distance of the foot of the ladder from the building.
Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
In an isosceles triangle ABC, AB = AC = 25 cm, BC = 14 cm. Calculate the altitude from A on BC.
The foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its tip reach?
Two poles of height 9 m and 14 m stand on a plane ground. If the distance between their feet is 12 m, find the distance between their tops.
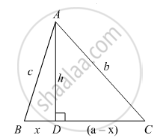
Using Pythagoras theorem determine the length of AD in terms of b and c shown in Figure.
A triangle has sides 5 cm, 12 cm and 13 cm. Find the length to one decimal place, of the perpendicular from the opposite vertex to the side whose length is 13 cm.
ABCD is a square. F is the mid-point of AB. BE is one third of BC. If the area of ΔFBE = 108 cm2, find the length of AC.
In an isosceles triangle ABC, if AB = AC = 13 cm and the altitude from A on BC is 5 cm, find BC.
In a ΔABC, AB = BC = CA = 2a and AD ⊥ BC. Prove that
(i) AD = a`sqrt3`
(ii) Area (ΔABC) = `sqrt3` a2
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
In the given figure, ∠B < 90° and segment AD ⊥ BC, show that
(i) b2 = h2 + a2 + x2 - 2ax
(ii) b2 = a2 + c2 - 2ax
In an equilateral ΔABC, AD ⊥ BC, prove that AD2 = 3BD2.
∆ABD is a right triangle right-angled at A and AC ⊥ BD. Show that
(i) AB2 = BC x BD
(ii) AC2 = BC x DC
(iii) AD2 = BD x CD
(iv) `"AB"^2/"AC"^2="BD"/"DC"`
A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
Determine whether the triangle having sides (a − 1) cm, 2`sqrta` cm and (a + 1) cm is a right-angled
triangle.
In an acute-angled triangle, express a median in terms of its sides.
In a quadrilateral ABCD, ∠B = 90°, AD2 = AB2 + BC2 + CD2, prove that ∠ACD = 90°.
In right-angled triangle ABC in which ∠C = 90°, if D is the mid-point of BC, prove that AB2 = 4AD2 − 3AC2.
In Figure, D is the mid-point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED
= x, AD = p and AE = h, prove that:
(i) `b^2 = p^2 + ax + a^2/4`
(ii) `c^2 = p^2 - ax + a^2/4`
(iii) `b^2 + c^2 = 2p^2 + a^2/2`
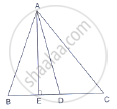
In ∆ABC, ∠A is obtuse, PB ⊥ AC and QC ⊥ AB. Prove that:
(i) AB ✕ AQ = AC ✕ AP
(ii) BC2 = (AC ✕ CP + AB ✕ BQ)
In a right ∆ABC right-angled at C, if D is the mid-point of BC, prove that BC2 = 4(AD2 − AC2).
An aeroplane leaves an airport and flies due north at a speed of 1000km/hr. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km/hr. How far apart will be the two planes after 1 hours?
RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.8 [Pages 123 - 128]
In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :

In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :

In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :
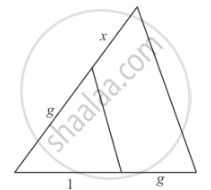
In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :

What values of x will make DE || AB in the given figure?

In ∆ABC, points P and Q are on CA and CB, respectively such that CA = 16 cm, CP = 10 cm, CB = 30 cm and CQ = 25 cm. Is PQ || AB?
In the given figure, DE || BD. Determine AC and AE.

In the given figure, given that ∆ABC ∼ ∆PQR and quad ABCD ∼ quad PQRS. Determine the value of x, y, z in each case.
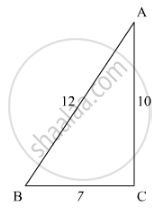
In the given figure, given that ∆ABC ∼ ∆PQR and quad ABCD ∼ quad PQRS. Determine the value of x, y, z in each case.

In ∆ABC, P and Q are points on sides AB and AC respectively such that PQ || BC. If AP = 4 cm, PB = 6 cm and PQ = 3 cm, determine BC.
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
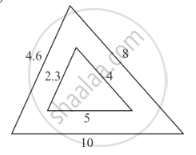
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.

In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.

In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
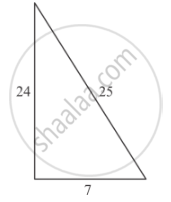
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
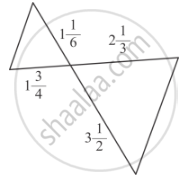
In ∆PQR, M and N are points on sides PQ and PR respectively such that PM = 15 cm and NR = 8 cm. If PQ = 25 cm and PR = 20 cm state whether MN || QR.
In ∆ABC, P and Q are points on sides AB and AC respectively such that PQ || BC. If AP = 3 cm, PB = 5 cm and AC = 8 cm, find AQ.
In the given figure, ∆AMB ∼ ∆CMD; determine MD in terms of x, y and z.

In ∆ABC, the bisector of ∠A intersects BC in D. If AB = 18 cm, AC = 15 cm and BC = 22 cm, find BD.
In the given figure, l || m
(i) Name three pairs of similar triangles with proper correspondence; write similarities.
(ii) Prove that

In the given figure,

AB || DC prove that
(i) ∆DMU ∼ ∆BMV
In the given figure,

AB || DC prove that
DM × BV = BM ✕ DU
ABCD is a trapezium in which AB || DC. P and Q are points on sides AD and BC such that PQ || AB. If PD = 18, BQ = 35 and QC = 15, find AD.
In ∆ABC, D and E are points on sides AB and AC respectively such that AD ✕ EC = AE ✕ DB. Prove that DE || BC.
ABCD is a trapezium having AB || DC. Prove that O, the point of intersection of diagonals, divides the two diagonals in the same ratio. Also prove that
Corresponding sides of two triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, determine the area of the larger triangle.
The area of two similar triangles are 36 cm2 and 100 cm2. If the length of a side of the smaller triangle in 3 cm, find the length of the corresponding side of the larger triangle.
Corresponding sides of two similar triangles are in the ratio 1 : 3. If the area of the smaller triangle in 40 cm2, find the area of the larger triangle.
In the given figure, each of PA, QB, RC and SD is perpendicular to l. If AB = 6 cm, BC = 9 cm, CD = 12 cm and PS = 36 cm, then determine PQ, QR and RS.

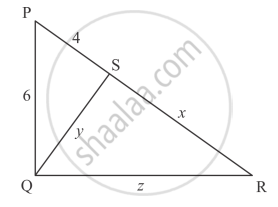
In each of the figures given below, an altitude is drawn to the hypotenuse by a right-angled triangle. The length of different line-segment are marked in each figure. Determine x, y, z in each case.
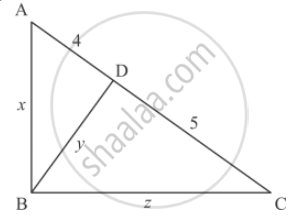
In each of the figures given below, an altitude is drawn to the hypotenuse by a right-angled triangle. The length of different line-segment are marked in each figure. Determine x, y, z in each case.
Prove that in an equilateral triangle, three times the square of a side is equal to four times the square of its altitudes.
In ∆ABC, AD and BE are altitude. Prove that\[\frac{ar\left( ∆ DEC \right)}{ar\left( ∆ ABC \right)} = \frac{{DC}^2}{{AC}^2}\]
The diagonals of quadrilateral ABCD intersect at O. Prove that
`[A(∆"ACB")]/[A(∆"ACD")] = "BO"/"DO"`
In ∆ABC, ray AD bisects ∠A and intersects BC in D. If BC = a, AC = b and AC = c, prove that \[BD = \frac{ac}{b + c}\]
In ∆ABC, ray AD bisects ∠A and intersects BC in D. If BC = a, AC = b and AC = c, prove that \[DC = \frac{ab}{b + c}\]
There is a staircase as shown in the given figure, connecting points A and B. Measurements of steps are marked in the figure. Find the straight line distance between A and B.

In ∆ABC, ∠A = 60°. Prove that BC2 = AB2 + AC2 − AB . AC.
In ∆ABC, ∠C is an obtuse angle. AD ⊥ BC and AB2 = AC2 + 3 BC2. Prove that BC = CD.
A point D is on the side BC of an equilateral triangle ABC such that\[DC = \frac{1}{4}BC\]. Prove that AD2 = 13 CD2.
In ∆ABC, if BD ⊥ AC and BC2 = 2 AC . CD, then prove that AB = AC.
In a quadrilateral ABCD, given that ∠A + ∠D = 90°. Prove that AC2 + BD2 = AD2 + BC2.
In ∆ABC, given that AB = AC and BD ⊥ AC. Prove that BC2 = 2 AC. CD
ABCD is a rectangle. Points M and N are on BD such that AM ⊥ BD and CN ⊥ BD. Prove that BM2 + BN2 = DM2 + DN2.
In ∆ABC, AD is a median. Prove that AB2 + AC2 = 2AD2 + 2DC2.
In ∆ABC, ∠ABC = 135°. Prove that AC2 = AB2 + BC2 + 4 ar (∆ABC)
In a quadrilateral ABCD, ∠B = 90°. If AD2 = AB2 + BC2 + CD2 then prove that ∠ACD = 90°.
In a triangle ABC, N is a point on AC such that BN ⊥ AC. If BN2 = AN . NC, prove that ∠B = 90°.
Nazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the road. Assuming that her string (from the tip of her road to the fly) is taut, how much string does she have out (in the given figure)? If she pulls the string at the rate of 5 cm per second, what will the horizontal distance of the fly from her after 12 seconds.

RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.9 [Pages 128 - 130]
State basic proportionality theorem and its converse.
In the adjoining figure, find AC.
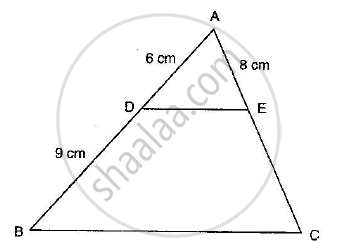
In the adjoining figure, if AD is the bisector of ∠A, what is AC?

State AAA similarity criterion.
State SSS similarity criterion.
State SAS similarity criterion.
In the adjoining figure, DE is parallel to BC and AD = 1 cm, BD = 2 cm. What is the ratio of the area of ∆ABC to the area of ∆ADE?
In the figure given below DE || BC. If AD = 2.4 cm, DB = 3.6 cm, AC = 5 cm. Find AE.
If the areas of two similar triangles ABC and PQR are in the ratio 9 : 16 and BC = 4.5 cm, what is the length of QR?
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, what is the length of the longest side of the smaller triangle?
If ABC and DEF are similar triangles such that ∠A = 57° and ∠E = 73°, what is the measure of ∠C?
If the altitude of two similar triangles are in the ratio 2 : 3, what is the ratio of their areas?
If ∆ABC and ∆DEF are two triangles such that\[\frac{AB}{DE} = \frac{BC}{EF} = \frac{CA}{FD} = \frac{3}{4}\], then write Area (∆ABC) : Area (∆DEF)
If ∆ABC and ∆DEF are similar triangles such that AB = 3 cm, BC = 2 cm, CA = 2.5 cm and EF = 4 cm, write the perimeter of ∆DEF.
State Pythagoras theorem and its converse.
The lengths of the diagonals of a rhombus are 30 cm and 40 cm. Find the side of the rhombus.
In the given figure, PQ || BC and AP : PB = 1 : 2. Find\[\frac{area \left( ∆ APQ \right)}{area \left( ∆ ABC \right)}\]

In the given figure, S and T are points on the sides PQ and PR respectively of ∆PQR such that PT = 2 cm, TR = 4 cm and ST is parallel to QR. Find the ratio of the areas of ∆PST and ∆PQR.
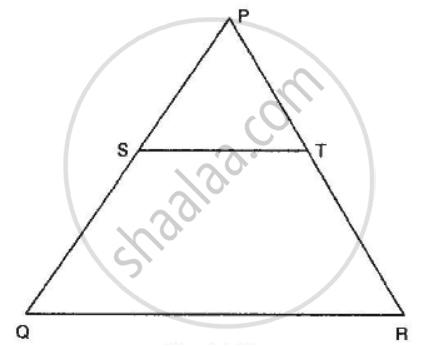
In the given figure, ∆AHK is similar to ∆ABC. If AK = 10 cm, BC = 3.5 cm and HK = 7 cm, find AC.

In the given figure, DE || BC in ∆ABC such that BC = 8 cm, AB = 6 cm and DA = 1.5 cm. Find DE.

In the given figure, DE || BC and \[AD = \frac{1}{2}BD\]. If BC = 4.5 cm, find DE.
In the given figure, LM = LN = 46°. Express x in terms of a, b and c where a, b, c are lengths of LM, MN and NK respectively.

RD Sharma solutions for Mathematics [English] Class 10 7 Triangles Exercise 7.10 [Pages 131 - 136]
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio.
2 : 3
4 : 9
81 : 16
16 : 81
The areas of two similar triangles are in respectively 9 cm2 and 16 cm2. The ratio of their corresponding sides is ______.
3 : 4
4 : 3
2 : 3
4 : 5
The areas of two similar triangles ∆ABC and ∆DEF are 144 cm2 and 81 cm2 respectively. If the longest side of larger ∆ABC be 36 cm, then the longest side of the smaller triangle ∆DEF is
20 cm
26 cm
27 cm
30 cm
∆ABC and ∆BDE are two equilateral triangles such that D is the mid-point of BC. The ratio of the areas of triangle ABC and BDE is
2 : 1
1 : 2
4 : 1
1 : 4
If ∆ABC and ∆DEF are similar such that 2AB = DE and BC = 8 cm, then EF =
16 cm
12 cm
8 cm
4 cm
If ∆ABC and ∆DEF are two triangles such tha\[\frac{AB}{DE} = \frac{BC}{EF} = \frac{CA}{FD} = \frac{2}{5}\] , then Area (∆ABC) : Area (∆DEF) =
2:5
4 : 25
4 : 15
8 : 125
XY is drawn parallel to the base BC of a ∆ABC cutting AB at X and AC at Y. If AB = 4 BX and YC = 2 cm, then AY =
2 cm
4 cm
6 cm
8 cm
Two poles of height 6 m and 11 m stand vertically upright on a plane ground. If the distance between their foot is 12 m, the distance between their tops is
12 m
14 m
13 m
11 m
In ∆ABC, D and E are points on side AB and AC respectively such that DE || BC and AD : DB = 3 : 1. If EA = 3.3 cm, then AC =
1.1 cm
4 cm
4.4 cm
5.5 cm
In triangles ABC and DEF, ∠A = ∠E = 40°, AB : ED = AC : EF and ∠F = 65°, then ∠B =
35°
65°
75°
85°
If ABC and DEF are similar triangles such that ∠A = 47° and ∠E = 83°, then ∠C =
50°
60°
70°
80°
If D, E, F are the mid-points of sides BC, CA and AB respectively of ∆ABC, then the ratio of the areas of triangles DEF and ABC is
1 : 4
1 : 2
2 : 3
4 : 5
In a ∆ABC, ∠A = 90°, AB = 5 cm and AC = 12 cm. If AD ⊥ BC, then AD =
\[\frac{13}{2}cm\]
- \[\frac{60}{13}cm\]
- \[\frac{13}{60}cm\]
- \[\frac{2\sqrt{15}}{13}cm\]
If ∆ABC is an equilateral triangle such that AD ⊥ BC, then AD2 =
- \[\frac{3}{2} {DC}^2\]
2 DC2
3 CD2
4 DC2
In a ∆ABC, AD is the bisector of ∠BAC. If AB = 6 cm, AC = 5 cm and BD = 3 cm, then DC =
11.3 cm
2.5 cm
3 : 5 cm
None of these
In a ∆ABC, AD is the bisector of ∠BAC. If AB = 8 cm, BD = 6 cm and DC = 3 cm. Find AC
4 cm
6 cm
3 cm
8 cm
ABCD is a trapezium such that BC || AD and AD = 4 cm. If the diagonals AC and BD intersect at O such that \[\frac{AO}{OC} = \frac{DO}{OB} = \frac{1}{2}\], then BC =
7 cm
8 cm
9 cm
6 cm
If ABC is a right triangle right-angled at B and M, N are the mid-points of AB and BC respectively, then 4(AN2 + CM2) =
4 AC2
5 AC2
- \[\frac{5}{4} {AC}^2\]
6 AC2
If in ∆ABC and ∆DEF, \[\frac{AB}{DE} = \frac{BC}{FD}\], then ∆ABC ∼ ∆DEF when
∠A = ∠F
∠A = ∠D
∠B = ∠D
∠B = ∠E
If in two triangles ABC and DEF, \[\frac{AB}{DE} = \frac{BC}{FE} = \frac{CA}{FD}\], then
∆FDE ∼ ∆CAB
∆FDE ∼ ∆ABC
∆CBA ∼ ∆FDE
∆BCA ∼ ∆FDE
∆ABC ∼ ∆DEF, ar(∆ABC) = 9 cm2, ar(∆DEF) = 16 cm2. If BC = 2.1 cm, then the measure of EF is
2.8 cm
4.2 cm
2.5 cm
4.1 cm
The length of the hypotenuse of an isosceles right triangle whose one side is\[4\sqrt{2} cm\]
12 cm
8 cm
- \[8\sqrt{2} cm\]
- \[12\sqrt{2} cm\]
In a ∆ABC, perpendicular AD from A and BC meets BC at D. If BD = 8 cm, DC = 2 cm and AD = 4 cm, then
∆ABC is isosceles
∆ABC is equilateral
AC = 2AB
∆ABC is right-angled at A
A man goes 24 m due west and then 7 m due north. How far is he from the starting point?
31 m
17 m
25 m
26 m
∆ABC ∼ ∆DEF. If BC = 3 cm, EF = 4 cm and ar(∆ABC) = 54 cm2, then ar(∆DEF) =
108 cm2
96 cm2
48 cm2
100 cm2
∆ABC ∼ ∆PQR such that ar(∆ABC) = 4 ar(∆PQR). If BC = 12 cm, then QR =
9 cm
10 cm
6 cm
8 cm
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, then the corresponding median of the other triangle is
11 cm
8.8 cm
11.1 cm
8.1 cm
In an equilateral triangle ABC if AD ⊥ BC, then AD2 =
CD2
2CD2
3CD2
4CD2
In an equilateral triangle ABC if AD ⊥ BC, then
5AB2 = 4AD2
3AB2 = 4AD2
4AB2 = 3AD2
2AB2 = 3AD2
∆ABC is an isosceles triangle in which ∠C = 90. If AC = 6 cm, then AB =
- \[6\sqrt{2} cm\]
6 cm
- \[2\sqrt{6} cm\]
- \[4\sqrt{2} cm\]
If in two triangle ABC and DEF, ∠A = ∠E, ∠B = ∠F, then which of the following is not true?
(a)\[\frac{BC}{DF} = \frac{AC}{DE}\]
(b)\[\frac{AB}{DE} = \frac{BC}{DF}\]
(c)\[\frac{AB}{EF} = \frac{AC}{DE}\]
(d)\[\frac{BC}{DF} = \frac{AB}{EF}\]
- \[\frac{BC}{DF} = \frac{AC}{DE}\]
- \[\frac{AB}{DE} = \frac{BC}{DF}\]
- \[\frac{AB}{EF} = \frac{AC}{DE}\]
- \[\frac{BC}{DF} = \frac{AB}{EF}\]
In the given figure the measure of ∠D and ∠F are respectively

50°, 40°
20°, 30°
40°, 50°
30°, 20°
In the given figure, the value of x for which DE || AB is

4
1
3
2
In the given figure, if ∠ADE = ∠ABC, then CE =
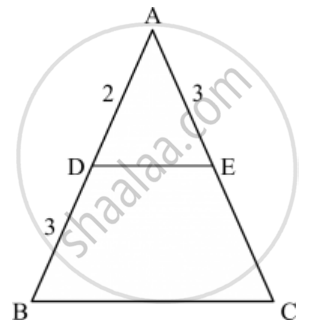
2
5
9/2
3
In the given figure, RS || DB || PQ. If CP = PD = 11 cm and DR = RA = 3 cm. Then the values of x and y are respectively.

12, 10
14, 6
10, 7
16, 8
In the given figure, if PB || CF and DP || EF, then \[\frac{AD}{DE} =\]
- \[\frac{3}{4}\]
- \[\frac{1}{3}\]
- \[\frac{1}{4}\]
- \[\frac{2}{3}\]
A chord of a circle of radius 10 cm subtends a right angle at the centre. The length of the chord (in cm) is
- \[5\sqrt{2}\]
- \[10\sqrt{2}\]
- \[\frac{5}{\sqrt{2}}\]
- \[10\sqrt{3}\]
A vertical stick 20 m long casts a shadow 10 m long on the ground. At the same time, a tower casts a shadow 50 m long on the ground. The height of the tower is
100 m
120 m
25 m
200 m
Two isosceles triangles have equal angles and their areas are in the ratio 16 : 25. The ratio of their corresponding heights is
4 : 5
5 : 4
3 : 2
5 : 7
∆ABC is such that AB = 3 cm, BC = 2 cm and CA = 2.5 cm. If ∆DEF ∼ ∆ABC and EF = 4 cm, then perimeter of ∆DEF is
7.5 cm
15 cm
22.5 cm
30 cm
In ∆ABC, a line XY parallel to BC cuts AB at X and AC at Y. If BY bisects ∠XYC, then
BC = CY
BC = BY
BC ≠ CY
BC ≠ BY
In a ∆ABC, ∠A = 90°, AB = 5 cm and AC = 12 cm. If AD ⊥ BC, then AD =
- \[\frac{13}{2}cm\]
- \[\frac{60}{13}cm\]
- \[\frac{13}{60}cm\]
- \[\frac{2\sqrt{15}}{13}cm\]
In a ∆ABC, point D is on side AB and point E is on side AC, such that BCED is a trapezium. If DE : BC = 3 : 5, then Area (∆ ADE) : Area (◻BCED) =
3 : 4
9 : 16
3 : 5
9 : 25
If ABC is an isosceles triangle and D is a point of BC such that AD ⊥ BC, then
AB2 − AD2 = BD.DC
AB2 − AD2 = BD2 − DC2
AB2 + AD2 = BD.DC
AB2 + AD2 = BD2 − DC2
∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
- \[\left( \frac{AB}{AC} \right)^2\]
- \[\frac{AB}{AC}\]
- \[\left( \frac{AB}{AD} \right)^2\]
- \[\frac{AB}{AD}\]
Non of the above
If E is a point on side CA of an equilateral triangle ABC such that BE ⊥ CA, then AB2 + BC2 + CA2 =
2 BE2
3 BE2
4 BE2
6 BE2
In a right triangle ABC right-angled at B, if P and Q are points on the sides AB and AC respectively, then
AQ2 + CP2 = 2(AC2 + PQ2)
2(AQ2 + CP2) = AC2 + PQ2
AQ2 + CP2 = AC2 + PQ2
\[AQ + CP = \frac{1}{2}\left( AC + PQ \right)\]
If ∆ABC ∼ ∆DEF such that DE = 3 cm, EF = 2 cm, DF = 2.5 cm, BC = 4 cm, then perimeter of ∆ABC is
18 cm
20 cm
12 cm
15 cm
If ∆ABC ∼ ∆DEF such that AB = 9.1 cm and DE = 6.5 cm. If the perimeter of ∆DEF is 25 cm, then the perimeter of ∆ABC is
36 cm
30 cm
34 cm
35 cm
In an isosceles triangle ABC if AC = BC and AB2 = 2AC2, then ∠C =
30°
45°
90°
60°
Solutions for 7: Triangles
![RD Sharma solutions for Mathematics [English] Class 10 chapter 7 - Triangles RD Sharma solutions for Mathematics [English] Class 10 chapter 7 - Triangles - Shaalaa.com](/images/8193647920-mathematics-english-class-10_6:5809898a5fef45e9a2f7e6b414d392fa.jpg)
RD Sharma solutions for Mathematics [English] Class 10 chapter 7 - Triangles
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 10 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. RD Sharma solutions for Mathematics Mathematics [English] Class 10 CBSE 7 (Triangles) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. RD Sharma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 10 chapter 7 Triangles are Properties of Ratios of Areas of Two Triangles, Similar Triangles, Areas of Similar Triangles, Basic Proportionality Theorem (Thales Theorem), Property of an Angle Bisector of a Triangle, Property of Three Parallel Lines and Their Transversals, Converse of Basic Proportionality Theorem, Similarity of Triangles, Criteria for Similarity of Triangles, Similar Figures, Similarity of Triangles, Basic Proportionality Theorem (Thales Theorem), Criteria for Similarity of Triangles, Areas of Similar Triangles, Triangles Examples and Solutions, Right-angled Triangles and Pythagoras Property, Similarity of Triangles, Similarity of Triangles, Application of Pythagoras Theorem in Acute Angle and Obtuse Angle, Ratio of Sides of Triangle, Concept of Angle Bisector.
Using RD Sharma Mathematics [English] Class 10 solutions Triangles exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in RD Sharma Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 10 students prefer RD Sharma Textbook Solutions to score more in exams.
Get the free view of Chapter 7, Triangles Mathematics [English] Class 10 additional questions for Mathematics Mathematics [English] Class 10 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
