Advertisements
Advertisements
प्रश्न
Calculate the height of an equilateral triangle each of whose sides measures 12 cm.
उत्तर
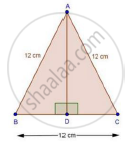
We have,
ΔABC is an equilateral Δ with side 12 cm.
Draw AE ⊥ BC
In ΔABD and ΔACD
∠ADB = ∠ADC [Each 90°]
AB = AC [Each 12 cm]
AD = AD [Common]
Then, ΔABD ≅ ΔACD [By RHS condition]
∴ AD2 + BD2 = AB2
⇒ AD2 + 62 = 122
⇒ AD2 = 144 − 36 = 108
⇒ AD = `sqrt`108 = 10.39 cm
APPEARS IN
संबंधित प्रश्न
If the sides of a triangle are 3 cm, 4 cm, and 6 cm long, determine whether the triangle is a right-angled triangle.
A man goes 15 metres due west and then 8 metres due north. How far is he from the starting point?
The lengths of the diagonals of a rhombus are 24 cm and 10 cm. Find each side of the rhombus.
Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm find the length of the other diagonal.
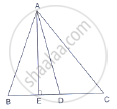
In Figure, D is the mid-point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED
= x, AD = p and AE = h, prove that:
(i) `b^2 = p^2 + ax + a^2/4`
(ii) `c^2 = p^2 - ax + a^2/4`
(iii) `b^2 + c^2 = 2p^2 + a^2/2`
In a quadrilateral ABCD, ∠B = 90°, AD2 = AB2 + BC2 + CD2, prove that ∠ACD = 90°.
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm ?
From given figure, In ∆ABC, AB ⊥ BC, AB = BC, AC = `5sqrt(2)` , then what is the height of ∆ABC?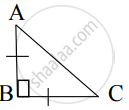
A girl walks 200m towards East and then 150m towards North. The distance of the girl from the starting point is ______.
In a ΔABC, ∠CAB is an obtuse angle. P is the circumcentre of ∆ABC. Prove that ∠CAB – ∠PBC = 90°.
