Advertisements
Advertisements
प्रश्न
If ∆ABC is an equilateral triangle such that AD ⊥ BC, then AD2 =
विकल्प
- \[\frac{3}{2} {DC}^2\]
2 DC2
3 CD2
4 DC2
उत्तर
Given: In an equilateral ΔABC, `AD ⊥ BC`.
Since `AD ⊥ BC`., BD = CD = \[\frac{BC}{2}\]
Applying Pythagoras theorem,
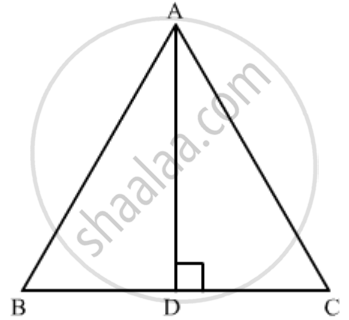
In ΔADC
`AC^2+AD^2+DC^2`
`BC^2=AD^2+DC^2`(Since AC=BC)
`(2DC)^2=AD^2+DC^2`(Since BC=2DC)
`4DC^2=AD^2+DC^2`
`3DC^2=AD^2`
`3DC^2=AD^2`
We got the result as `c`
APPEARS IN
संबंधित प्रश्न
the below given figure, a triangle ABC is drawn to circumscribe a circle of radius 3 cm, such that the segments BD and DC are respectively of lengths 6 cm and 9 cm. If the area of ΔABC is 54 cm2, then find the lengths of sides AB and AC.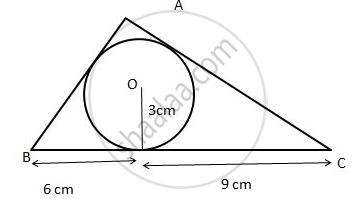
A point D is on the side BC of an equilateral triangle ABC such that\[DC = \frac{1}{4}BC\]. Prove that AD2 = 13 CD2.
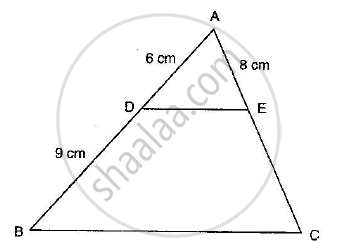
In the adjoining figure, find AC.
In the adjoining figure, if AD is the bisector of ∠A, what is AC?

If ∆ABC and ∆DEF are similar triangles such that AB = 3 cm, BC = 2 cm, CA = 2.5 cm and EF = 4 cm, write the perimeter of ∆DEF.
If ABC is a right triangle right-angled at B and M, N are the mid-points of AB and BC respectively, then 4(AN2 + CM2) =
∆ABC ∼ ∆DEF. If BC = 3 cm, EF = 4 cm and ar(∆ABC) = 54 cm2, then ar(∆DEF) =
∆ABC ∼ ∆PQR such that ar(∆ABC) = 4 ar(∆PQR). If BC = 12 cm, then QR =
In a ∆ABC, point D is on side AB and point E is on side AC, such that BCED is a trapezium. If DE : BC = 3 : 5, then Area (∆ ADE) : Area (◻BCED) =
If ABC is an isosceles triangle and D is a point of BC such that AD ⊥ BC, then
