Advertisements
Advertisements
प्रश्न
If ABC is a right triangle right-angled at B and M, N are the mid-points of AB and BC respectively, then 4(AN2 + CM2) =
विकल्प
4 AC2
5 AC2
- \[\frac{5}{4} {AC}^2\]
6 AC2
उत्तर
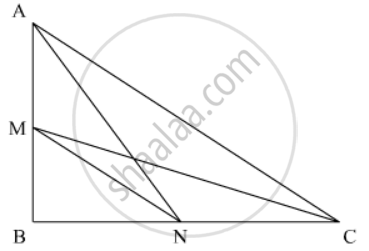
M is the mid-point of AB.
∴ \[BM = \frac{AB}{2}\]
N is the mid-point of BC.
∴\[BN = \frac{BC}{2}\]
Now,
`AN^2+CM^2=(AB^2+(BC)^2)+((AB)^2+BC^2)`
`=AB^2+BC^2+1/4AB^2+BC^2`
`=5/4(AB^2+BC^2)`
`⇒ 4(AN^2+CM^2)=5AC^2`
Hence option (b) is correct.
APPEARS IN
संबंधित प्रश्न
A vertical stick of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AB = 10.8cm, AD = 6.3cm, AC = 9.6cm and EC = 4cm.

What values of x will make DE || AB in the given figure?

In the given figure, given that ∆ABC ∼ ∆PQR and quad ABCD ∼ quad PQRS. Determine the value of x, y, z in each case.
In the given figure, ∆AMB ∼ ∆CMD; determine MD in terms of x, y and z.

The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, what is the length of the longest side of the smaller triangle?
If ∆ABC and ∆DEF are similar such that 2AB = DE and BC = 8 cm, then EF =
XY is drawn parallel to the base BC of a ∆ABC cutting AB at X and AC at Y. If AB = 4 BX and YC = 2 cm, then AY =
In triangles ABC and DEF, ∠A = ∠E = 40°, AB : ED = AC : EF and ∠F = 65°, then ∠B =
In a right triangle ABC right-angled at B, if P and Q are points on the sides AB and AC respectively, then
