Advertisements
Advertisements
प्रश्न
In the given figure, ∆AMB ∼ ∆CMD; determine MD in terms of x, y and z.

उत्तर
We are given ∆AMB ∼ ∆CMD
We have to determine the value of MD in terms of x, y and z.
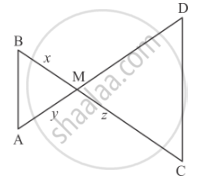
Given `Δ AMB ∼ Δ CMD `
\[\Rightarrow \frac{BM}{MD} = \frac{AM}{CM}\]
\[\frac{x}{MD} = \frac{y}{z}\]
By cross multiplication we get `MD = (xz)/y`
Hence, the value of MD is `(xz)/y`.
APPEARS IN
संबंधित प्रश्न
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AD = 7.2cm, AE = 6.4cm, AB = 12cm and AC = 10cm.
A ladder is placed in such a way that its foot is at a distance of 15m from a wall and its top reaches a window 20m above the ground. Find the length of the ladder.
What values of x will make DE || AB in the given figure?

Nazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the road. Assuming that her string (from the tip of her road to the fly) is taut, how much string does she have out (in the given figure)? If she pulls the string at the rate of 5 cm per second, what will the horizontal distance of the fly from her after 12 seconds.

In the figure given below DE || BC. If AD = 2.4 cm, DB = 3.6 cm, AC = 5 cm. Find AE.
XY is drawn parallel to the base BC of a ∆ABC cutting AB at X and AC at Y. If AB = 4 BX and YC = 2 cm, then AY =
If D, E, F are the mid-points of sides BC, CA and AB respectively of ∆ABC, then the ratio of the areas of triangles DEF and ABC is
A man goes 24 m due west and then 7 m due north. How far is he from the starting point?
∆ABC ∼ ∆PQR such that ar(∆ABC) = 4 ar(∆PQR). If BC = 12 cm, then QR =
If E is a point on side CA of an equilateral triangle ABC such that BE ⊥ CA, then AB2 + BC2 + CA2 =
