Advertisements
Advertisements
प्रश्न
If in two triangle ABC and DEF, ∠A = ∠E, ∠B = ∠F, then which of the following is not true?
(a)\[\frac{BC}{DF} = \frac{AC}{DE}\]
(b)\[\frac{AB}{DE} = \frac{BC}{DF}\]
(c)\[\frac{AB}{EF} = \frac{AC}{DE}\]
(d)\[\frac{BC}{DF} = \frac{AB}{EF}\]
विकल्प
- \[\frac{BC}{DF} = \frac{AC}{DE}\]
- \[\frac{AB}{DE} = \frac{BC}{DF}\]
- \[\frac{AB}{EF} = \frac{AC}{DE}\]
- \[\frac{BC}{DF} = \frac{AB}{EF}\]
उत्तर

In ΔABC and ΔDEF
`∠ A = ∠ E`
`∠ B = ∠ F`
∴ ΔABC and ΔDEF are similar triangles.
Hence `(AB)/(EF)=(BC)/(FD)=(CA)/(DE)`
Hence the correct answer is (b).
APPEARS IN
संबंधित प्रश्न
In below figure, If AB || CD, find the value of x.

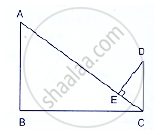
In Figure below, if AB ⊥ BC, DC ⊥ BC and DE ⊥ AC, Prove that Δ CED ~ ABC.
In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :

In the given figure, given that ∆ABC ∼ ∆PQR and quad ABCD ∼ quad PQRS. Determine the value of x, y, z in each case.

Corresponding sides of two triangles are in the ratio 2 : 3. If the area of the smaller triangle is 48 cm2, determine the area of the larger triangle.
State SAS similarity criterion.
In the given figure, DE || BC in ∆ABC such that BC = 8 cm, AB = 6 cm and DA = 1.5 cm. Find DE.

In ∆ABC, a line XY parallel to BC cuts AB at X and AC at Y. If BY bisects ∠XYC, then
In a ∆ABC, point D is on side AB and point E is on side AC, such that BCED is a trapezium. If DE : BC = 3 : 5, then Area (∆ ADE) : Area (◻BCED) =
In a right triangle ABC right-angled at B, if P and Q are points on the sides AB and AC respectively, then
