Advertisements
Advertisements
प्रश्न
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, then the corresponding median of the other triangle is
विकल्प
11 cm
8.8 cm
11.1 cm
8.1 cm
उत्तर
Given: The area of two similar triangles is 121cm2 and 64cm2 respectively. The median of the first triangle is 2.1cm.
To find: Corresponding medians of the other triangle
We know that the ratio of areas of two similar triangles is equal to the ratio of squares of their medians.
`\text{ar(triangle 1)}/\text{ar(triangle 1)}=((\text{median} 1^2)/(\text{median2}^2))^2`
`121/64=((12.1)/\text{median2})^2`
Taking square root on both side, we get
\[ \Rightarrow median2 = 8 . 8 cm\]
Hence the correct answer is `b`.
APPEARS IN
संबंधित प्रश्न
the below given figure, a triangle ABC is drawn to circumscribe a circle of radius 3 cm, such that the segments BD and DC are respectively of lengths 6 cm and 9 cm. If the area of ΔABC is 54 cm2, then find the lengths of sides AB and AC.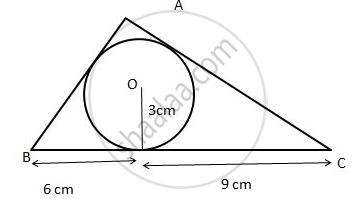
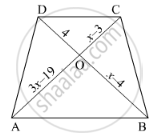
In below figure, AB || CD. If OA = 3x – 19, OB = x – 4, OC = x – 3 and OD = 4, find x.
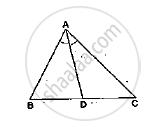
In a ΔABC, AD is the bisector of ∠A.
If AB = 6.4cm, AC = 8cm and BD = 5.6cm, find DC.
In each of the figures [(i)-(iv)] given below, a line segment is drawn parallel to one side of the triangle and the lengths of certain line-segment are marked. Find the value of x in each of the following :

The diagonals of quadrilateral ABCD intersect at O. Prove that
`[A(∆"ACB")]/[A(∆"ACD")] = "BO"/"DO"`
In ∆ABC, ray AD bisects ∠A and intersects BC in D. If BC = a, AC = b and AC = c, prove that \[DC = \frac{ab}{b + c}\]
State SSS similarity criterion.
In the given figure, RS || DB || PQ. If CP = PD = 11 cm and DR = RA = 3 cm. Then the values of x and y are respectively.

In ∆ABC, a line XY parallel to BC cuts AB at X and AC at Y. If BY bisects ∠XYC, then
In a ∆ABC, ∠A = 90°, AB = 5 cm and AC = 12 cm. If AD ⊥ BC, then AD =
