Advertisements
Advertisements
प्रश्न
In the given figure, RS || DB || PQ. If CP = PD = 11 cm and DR = RA = 3 cm. Then the values of x and y are respectively.

विकल्प
12, 10
14, 6
10, 7
16, 8
उत्तर
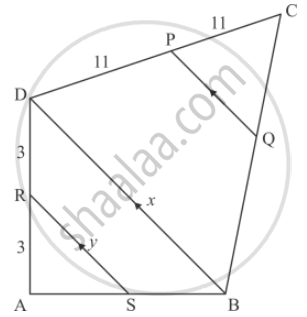
Given: RS || DB || PQ. CP = PD = 11cm and DR = RA = 3cm
To find: the value of x and y respectively.
\[In ∆ ASR and ∆ ABD, \]
\[\angle ASR = \angle ABQ \left( \text{Corresponding angles} \right)\]
\[\angle A = \angle A \left( \text{Common} \right)\]
\[ \therefore ∆ ASR ~ ∆ ABD \left( AA \hspace{0.167em} \text{Similarity} \right)\]
`(AR)/(AD)=(AS)/(AB)=(RS)/(DB)`
`3/6=(RS)/(DB)`
`1/2=x/y`
`x=2y`
This relation is satisfied by option (d).
Hence, x = 16 cm and y = 8cm
Hence the result is `d`
APPEARS IN
संबंधित प्रश्न
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AB = 11.7cm, AC = 11.2cm, BD = 6.5cm and AE = 4.2cm.
What values of x will make DE || AB in the given figure?

In the given figure, given that ∆ABC ∼ ∆PQR and quad ABCD ∼ quad PQRS. Determine the value of x, y, z in each case.

In the given figure, ∆AMB ∼ ∆CMD; determine MD in terms of x, y and z.

In ∆ABC, if BD ⊥ AC and BC2 = 2 AC . CD, then prove that AB = AC.
ABCD is a rectangle. Points M and N are on BD such that AM ⊥ BD and CN ⊥ BD. Prove that BM2 + BN2 = DM2 + DN2.
In the given figure, ∆AHK is similar to ∆ABC. If AK = 10 cm, BC = 3.5 cm and HK = 7 cm, find AC.

In the given figure the measure of ∠D and ∠F are respectively

∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
If ∆ABC ∼ ∆DEF such that DE = 3 cm, EF = 2 cm, DF = 2.5 cm, BC = 4 cm, then perimeter of ∆ABC is
