Advertisements
Advertisements
प्रश्न
ABCD is a rectangle. Points M and N are on BD such that AM ⊥ BD and CN ⊥ BD. Prove that BM2 + BN2 = DM2 + DN2.
उत्तर
Given: A rectangle ABCD where AM ⊥ BD and CN ⊥ BD.
To prove: BM2 + BN2 = DM2 + DN2
Proof:
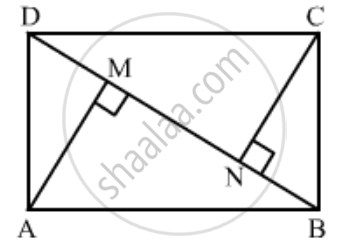
Apply Pythagoras Theorem in ΔAMB and ΔCND,
AB2 = AM2 + MB2
CD2 = CN2 + ND2
Since AB = CD, AM2 + MB2 = CN2 + ND2
⇒ AM2 − CN2 = ND2 − MB2 … (i)
Again apply Pythagoras Theorem in ΔAMD and ΔCNB,
AD2 = AM2 + MD2
CB2 = CN2 + NB2
Since AD = BC, AM2 + MD2 = CN2 + NB2
⇒ AM2 − CN2 = NB2 − MD2 … (ii)
Equating (i) and (ii),
ND2 − MB2 = NB2 − MD2
I.e., BM2 + BN2 = DM2 + DN2
This proves the given relation.
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral in which AD = BC. If P, Q, R, S be the mid-points of AB, AC, CD and BD respectively, show that PQRS is a rhombus.
A girl of height 90 cm is walking away from the base of a lamp-post at a speed of 1.2m/sec. If the lamp is 3.6 m above the ground, find the length of her shadow after 4 seconds.
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
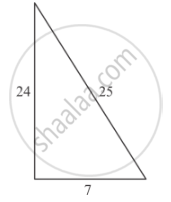
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
In a triangle ABC, N is a point on AC such that BN ⊥ AC. If BN2 = AN . NC, prove that ∠B = 90°.
State SSS similarity criterion.
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, what is the length of the longest side of the smaller triangle?
If the altitude of two similar triangles are in the ratio 2 : 3, what is the ratio of their areas?
In the given figure, if PB || CF and DP || EF, then \[\frac{AD}{DE} =\]
A chord of a circle of radius 10 cm subtends a right angle at the centre. The length of the chord (in cm) is
