Advertisements
Advertisements
Question
ABCD is a rectangle. Points M and N are on BD such that AM ⊥ BD and CN ⊥ BD. Prove that BM2 + BN2 = DM2 + DN2.
Solution
Given: A rectangle ABCD where AM ⊥ BD and CN ⊥ BD.
To prove: BM2 + BN2 = DM2 + DN2
Proof:
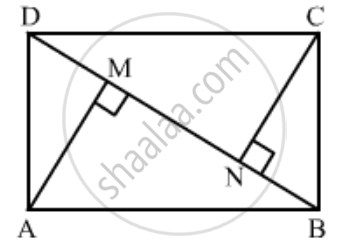
Apply Pythagoras Theorem in ΔAMB and ΔCND,
AB2 = AM2 + MB2
CD2 = CN2 + ND2
Since AB = CD, AM2 + MB2 = CN2 + ND2
⇒ AM2 − CN2 = ND2 − MB2 … (i)
Again apply Pythagoras Theorem in ΔAMD and ΔCNB,
AD2 = AM2 + MD2
CB2 = CN2 + NB2
Since AD = BC, AM2 + MD2 = CN2 + NB2
⇒ AM2 − CN2 = NB2 − MD2 … (ii)
Equating (i) and (ii),
ND2 − MB2 = NB2 − MD2
I.e., BM2 + BN2 = DM2 + DN2
This proves the given relation.
APPEARS IN
RELATED QUESTIONS
In the below figure, a triangle ABC is drawn to circumscribe a circle of radius 3 cm, such that the segments BD and DC are respectively of lengths 6 cm and 9 cm. If the area of Δ ABC is 54 cm2, then find the lengths of sides AB and AC.

In the below figure, If AB || CD, find the value of x.

In ∆PQR, M and N are points on sides PQ and PR respectively such that PM = 15 cm and NR = 8 cm. If PQ = 25 cm and PR = 20 cm state whether MN || QR.
In ∆ABC, ray AD bisects ∠A and intersects BC in D. If BC = a, AC = b and AC = c, prove that \[DC = \frac{ab}{b + c}\]
State SAS similarity criterion.
Sides of two similar triangles are in the ratio 4 : 9. Areas of these triangles are in the ratio.
If in ∆ABC and ∆DEF, \[\frac{AB}{DE} = \frac{BC}{FD}\], then ∆ABC ∼ ∆DEF when
A man goes 24 m due west and then 7 m due north. How far is he from the starting point?
In an equilateral triangle ABC if AD ⊥ BC, then
∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
