Advertisements
Advertisements
Question
If in ∆ABC and ∆DEF, \[\frac{AB}{DE} = \frac{BC}{FD}\], then ∆ABC ∼ ∆DEF when
Options
∠A = ∠F
∠A = ∠D
∠B = ∠D
∠B = ∠E
Solution
Given: In ΔABC and ΔDEF, `(AB)/(DE)=(BC)/(FD)`.
We know that if in two triangles, one pair of corresponding sides are proportional and the included angles are equal, then the two triangles are similar.
Then, `∠B=∠D`

Hence, ΔABC is similar to ΔDEF, we should have .`∠B=∠D`
Hence the correct answer is `c`
APPEARS IN
RELATED QUESTIONS
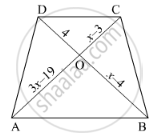
In below figure, AB || CD. If OA = 3x – 19, OB = x – 4, OC = x – 3 and OD = 4, find x.
In an isosceles ΔABC, the base AB is produced both the ways to P and Q such that AP × BQ = AC2. Prove that ΔAPC ~ ΔBCQ.
In ∆ABC, AD and BE are altitude. Prove that\[\frac{ar\left( ∆ DEC \right)}{ar\left( ∆ ABC \right)} = \frac{{DC}^2}{{AC}^2}\]
In ∆ABC, if BD ⊥ AC and BC2 = 2 AC . CD, then prove that AB = AC.
In the adjoining figure, if AD is the bisector of ∠A, what is AC?

∆ABC and ∆BDE are two equilateral triangles such that D is the mid-point of BC. The ratio of the areas of triangle ABC and BDE is
∆ABC ∼ ∆DEF, ar(∆ABC) = 9 cm2, ar(∆DEF) = 16 cm2. If BC = 2.1 cm, then the measure of EF is
In an equilateral triangle ABC if AD ⊥ BC, then
Two isosceles triangles have equal angles and their areas are in the ratio 16 : 25. The ratio of their corresponding heights is
∆ABC is such that AB = 3cm, BC = 2cm, CA = 2.5cm. If ∆ABC ~ ∆DEF and EF = 4cm, then perimeter of ∆DEF is ______.
