Advertisements
Advertisements
Question
In an isosceles ΔABC, the base AB is produced both the ways to P and Q such that AP × BQ = AC2. Prove that ΔAPC ~ ΔBCQ.
Solution
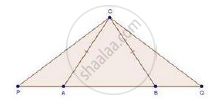
Given: In ΔABC, CA = CB and AP × BQ = AC2
To prove: ΔAPC ~ ΔBCQ
Proof:
AP × BQ = AC2 [Given]
⇒ AP × BQ = AC × AC
⇒ AP × BQ = AC × BC [AC = BC given]
`rArr"AP"/"BC"="AC"/"BQ"` ......(i)
Since, CA = CB [Given]
Then, ∠CAB = ∠CBA …(ii) [Opposite angles to equal sides]
Now, ∠CAB + ∠CAP = 180° …(iii) [Linear pair of angles]
And, ∠CBA + ∠CBQ = 180° …(iv) [Linear pair of angles]
Compare equation (ii) (iii) & (iv)
∠CAP = ∠CBQ …(v)
In ΔAPC and ΔBCQ
∠CAP = ∠CBQ [From (v)]
`"AP"/"BC"="AC"/"BQ"` [From (i)]
Then, ΔAPC~ΔBCQ [By SAS similarity]
APPEARS IN
RELATED QUESTIONS
In below figure, If AB || CD, find the value of x.

In the below figure, If AB || CD, find the value of x.

D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AB = 11.7cm, AC = 11.2cm, BD = 6.5cm and AE = 4.2cm.
In ∆ABC, points P and Q are on CA and CB, respectively such that CA = 16 cm, CP = 10 cm, CB = 30 cm and CQ = 25 cm. Is PQ || AB?
In the given figure, l || m
(i) Name three pairs of similar triangles with proper correspondence; write similarities.
(ii) Prove that

State Pythagoras theorem and its converse.
If D, E, F are the mid-points of sides BC, CA and AB respectively of ∆ABC, then the ratio of the areas of triangles DEF and ABC is
In the given figure, RS || DB || PQ. If CP = PD = 11 cm and DR = RA = 3 cm. Then the values of x and y are respectively.

A chord of a circle of radius 10 cm subtends a right angle at the centre. The length of the chord (in cm) is
In a ∆ABC, perpendicular AD from A and BC meets BC at D. If BD = 8 cm, DC = 2 cm and AD = 4 cm, then
