Question

If D, E, F are the mid-points of sides BC, CA and AB respectively of ∆ABC, then the ratio of the areas of triangles DEF and ABC is

Options

• 1 : 4

• 1 : 2

• 2 : 3

• 4 : 5

Answer 1

GIVEN: In ΔABC, D, E and F are the midpoints of BC, CA, and AB respectively.

TO FIND: Ratio of the areas of ΔDEF and ΔABC

Since it is given that D and, E are the midpoints of BC, and AC respectively.

Therefore DE || AB, DE || FA……(1)

Again it is given that D and, F are the midpoints of BC, and, AB respectively.

Therefore, DF || CA, DF || AE……(2)

From (1) and (2) we get AFDE is a parallelogram.

Similarly we can prove that BDEF is a parallelogram.

Now, in ΔADE and ΔABC

`∠FDE=∠A\text{(opposite angles of}||^(gm)AFDE)`

`∠DEF = ∠ B=\text{(opposite angles of}||^(gm)BDEF)`

`⇒ Δ ABC ∼ Δ DEF (\text{AA similarity criterion})`

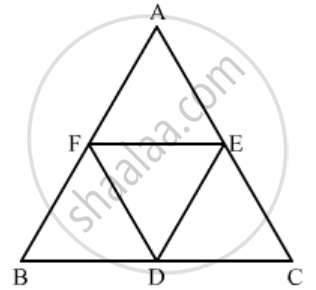
We know that the ratio of areas of two similar triangles is equal to the ratio of squares of their corresponding sides.

`{ar(Δ DEF)}/{ar(Δ ABC)}=((DE)/(AB))^2`

`{ar(Δ DEF)}/{ar(Δ ABC)}=((1/2(AB))/(AB))^2(Since DE =1/2AB)`

`{ar(Δ DEF)}/{ar(Δ ABC)}=(1/4)`

Hence the correct option is `a`