Advertisements
Advertisements
प्रश्न
In the given figure, each of PA, QB, RC and SD is perpendicular to l. If AB = 6 cm, BC = 9 cm, CD = 12 cm and PS = 36 cm, then determine PQ, QR and RS.

उत्तर
Given `AB = 6cm ,BC = 9cm,CD= 12cm,AD=27cm ` and `PS = 36cm`
PA, QB, RC and SD is perpendicular to l,
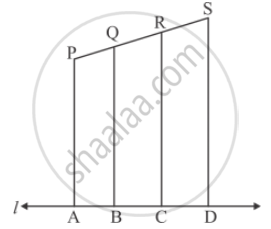
Therefore, by the corollory of basic proportionality theorem, we have
`(AB)/(AD)=(PQ)/(PS)`
`(BC)/(AD)=(QR)/(PS)`
`(CD)/(AD)=(RS)/(PS)`
`⇒ (AB)/(AD)=(PQ)/(PS)`
`6/27=(PQ)/36`
`(6xx36)/27=PQ`
`PQ = 8`
Now for QR
`(BC)/(AD)=(QR)/(PS)`
`(9)/(27)=(QR)/(36)`
`(6xx36)/27=QR`
`QR=12`
Again for RS
`(CD)/(AD)=(RS)/(PS)`
`12/27=(RS)/36`
`(12xx36)/27=RS`
`RS = 16`
Hence, the values of PQ, QR and RS are `8,12,16`respectively.
APPEARS IN
संबंधित प्रश्न
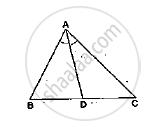
In a ΔABC, AD is the bisector of ∠A.
If AB = 6.4cm, AC = 8cm and BD = 5.6cm, find DC.
A 13m long ladder reaches a window of a building 12m above the ground. Determine the distance of the foot of the ladder from the building.
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
The diagonals of quadrilateral ABCD intersect at O. Prove that
`[A(∆"ACB")]/[A(∆"ACD")] = "BO"/"DO"`
In ∆ABC, ray AD bisects ∠A and intersects BC in D. If BC = a, AC = b and AC = c, prove that \[BD = \frac{ac}{b + c}\]
In ∆ABC, given that AB = AC and BD ⊥ AC. Prove that BC2 = 2 AC. CD
ABCD is a rectangle. Points M and N are on BD such that AM ⊥ BD and CN ⊥ BD. Prove that BM2 + BN2 = DM2 + DN2.
Nazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the road. Assuming that her string (from the tip of her road to the fly) is taut, how much string does she have out (in the given figure)? If she pulls the string at the rate of 5 cm per second, what will the horizontal distance of the fly from her after 12 seconds.

If ABC and DEF are similar triangles such that ∠A = 47° and ∠E = 83°, then ∠C =
In the given figure, if PB || CF and DP || EF, then \[\frac{AD}{DE} =\]
