Advertisements
Advertisements
प्रश्न
Two poles of height 6 m and 11 m stand vertically upright on a plane ground. If the distance between their foot is 12 m, the distance between their tops is
विकल्प
12 m
14 m
13 m
11 m
उत्तर
Given: Two poles of heights 6m and 11m stand vertically upright on a plane ground. Distance between their foot is 12 m.
To find: Distance between their tops.
Let CD be the pole with height 6m.
AB is the pole with height 11m, distance between their foot i.e. DB is 12 m.
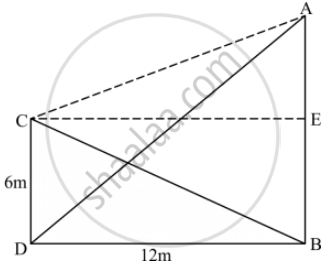
Let us assume a point E on the pole AB which is 6m from the base of AB.
Hence
AE = AB − 6 = 11 − 6 = 5 m
Now in right triangle AEC, Applying Pythagoras theorem
AC2 = AE2 + EC2
AC2 = 52 + 122 (since CDEB forms a rectangle and opposite sides of rectangle are equal)
AC2 = 25 + 144
AC2 = 169
`AC= 13cm`
Thus, the distance between their tops is 13m.
Hence correct answer is `C`.
APPEARS IN
संबंधित प्रश्न
D and E are points on the sides AB and AC respectively of a ΔABC. In each of the following cases, determine whether DE║BC or not.
AD = 7.2cm, AE = 6.4cm, AB = 12cm and AC = 10cm.
What values of x will make DE || AB in the given figure?

In ∆ABC, AD and BE are altitude. Prove that\[\frac{ar\left( ∆ DEC \right)}{ar\left( ∆ ABC \right)} = \frac{{DC}^2}{{AC}^2}\]
State SSS similarity criterion.
If ∆ABC and ∆DEF are two triangles such that\[\frac{AB}{DE} = \frac{BC}{EF} = \frac{CA}{FD} = \frac{3}{4}\], then write Area (∆ABC) : Area (∆DEF)
The lengths of the diagonals of a rhombus are 30 cm and 40 cm. Find the side of the rhombus.
XY is drawn parallel to the base BC of a ∆ABC cutting AB at X and AC at Y. If AB = 4 BX and YC = 2 cm, then AY =
In an equilateral triangle ABC if AD ⊥ BC, then AD2 =
∆ABC is an isosceles triangle in which ∠C = 90. If AC = 6 cm, then AB =
∆ABC is a right triangle right-angled at A and AD ⊥ BC. Then, \[\frac{BD}{DC} =\]
