Advertisements
Advertisements
प्रश्न
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
Advertisements
उत्तर
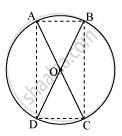
Let two chords AB and CD are intersecting each other at point O.
In ΔAOB and ΔCOD,
OA = OC (Given)
OB = OD (Given)
∠AOB = ∠COD (Vertically opposite angles)
ΔAOB ≅ ΔCOD (SAS congruence rule)
AB = CD (By CPCT)
Similarly, it can be proved that ΔAOD ≅ ΔCOB
∴ AD = CB (By CPCT)
Since in quadrilateral ACBD, opposite sides are equal in length, ACBD is a parallelogram.
We know that opposite angles of a parallelogram are equal.
∴ ∠A = ∠C
However, ∠A + ∠C = 180° (ABCD is a cyclic quadrilateral)
⇒ ∠A + ∠A = 180°
⇒ 2 ∠A = 180°
⇒ ∠A = 90°
As ACBD is a parallelogram and one of its interior angles is 90°, therefore, it is a rectangle.
∠A is the angle subtended by chord BD. And as ∠A = 90°, therefore, BD should be the diameter of the circle. Similarly, AC is the diameter of the circle.
संबंधित प्रश्न
In the given figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE = AD.
Two chords AB and CD of lengths 5 cm 11cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of the triangle DEF are 90°-A, 90° − `1/2 A, 90° − 1/2 B, 90° − 1/2` C.
In the figure, `square`ABCD is a cyclic quadrilateral. Seg AB is a diameter. If ∠ ADC = 120˚, complete the following activity to find measure of ∠ BAC.
`square` ABCD is a cyclic quadrilateral.
∴ ∠ ADC + ∠ ABC = 180°
∴ 120˚ + ∠ ABC = 180°
∴ ∠ ABC = ______
But ∠ ACB = ______ .......(angle in semicircle)
In Δ ABC,
∠ BAC + ∠ ACB + ∠ ABC = 180°
∴ ∠BAC + ______ = 180°
∴ ∠ BAC = ______
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.
ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
ABCD is a cyclic quadrilateral in ∠DBC = 80° and ∠BAC = 40°. Find ∠BCD.
Prove that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
