Advertisements
Advertisements
प्रश्न
|
In a GPS, The lines that run east-west are known as lines of latitude, and the lines running north-south are known as lines of longitude. The latitude and the longitude of a place are its coordinates and the distance formula is used to find the distance between two places. The distance between two parallel lines is approximately 150 km. A family from Uttar Pradesh planned a round trip from Lucknow (L) to Puri (P) via Bhuj (B) and Nashik (N) as shown in the given figure below.
|
Based on the above information answer the following questions using the coordinate geometry.
- Find the distance between Lucknow (L) to Bhuj (B).
- If Kota (K), internally divide the line segment joining Lucknow (L) to Bhuj (B) into 3 : 2 then find the coordinate of Kota (K).
- Name the type of triangle formed by the places Lucknow (L), Nashik (N) and Puri (P)
[OR]
Find a place (point) on the longitude (y-axis) which is equidistant from the points Lucknow (L) and Puri (P).
उत्तर
I. LB = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)`
⇒ LB = `sqrt((0 - 5)^2 + (7 - 10)^2)`
LB = `sqrt((5)^2 + (3)^2)`
⇒ LB = `sqrt(25 + 9)`
LB = `sqrt(34)`
Hence the distance is `150 sqrt(34)` km
II. Coordinate of Kota (K) is `((3 xx 5 + 2 xx 0)/(3 + 2), (3 xx 7 + 2 xx 10)/(3 + 2))`
= `((15 + 0)/5, (21 + 20)/5)`
= `(3, 41/5)`
III. L(5, 10), N(2, 6), P(8, 6)
LN = `sqrt((2 - 5)^2 + (6 - 10)^2)`
= `sqrt((3)^2 + (4)^2)`
= `sqrt(9 + 16)`
= `sqrt(25)`
= 5
NP = `sqrt((8 - 2)^2 + (6 - 6)^2)`
= `sqrt((4)^2 + (0)^2)`
= 4
PL = `sqrt((8 - 5)^2 + (6 - 10)^2)`
= `sqrt((3)^2 + (4)^2)`
⇒ LB = `sqrt(9 + 16)`
= `sqrt(25)`
= 5
As LN = PL ≠ NP, So ∆LNP is an isosceles triangle.
[OR]
Let A(0, b) be a point on the y-axis then AL = AP
⇒ `sqrt((5 - 0)^2 + (10 - b)^2) = sqrt((8 - 0)^2 + (6 - b)^2)`
⇒ (5)2 + (10 − b)2 = (8)2 + (6 − b)2
⇒ 25 + 100 − 20b + b2 = 64 + 36 − 12b + b2
⇒ 8b = 25
⇒ b = `25/8`
So, the coordinate on y axis is `(0, 25/8)`
APPEARS IN
संबंधित प्रश्न
Find a relation between x and y such that the point (x, y) is equidistant from the point (3, 6) and (−3, 4).
Given a triangle ABC in which A = (4, −4), B = (0, 5) and C = (5, 10). A point P lies on BC such that BP : PC = 3 : 2. Find the length of line segment AP.
Find the distance of the following points from the origin:
(iii) C (-4,-6)
AB and AC are the two chords of a circle whose radius is r. If p and q are
the distance of chord AB and CD, from the centre respectively and if
AB = 2AC then proove that 4q2 = p2 + 3r2.
Find the relation between a and b if the point P(a ,b) is equidistant from A (6,-1) and B (5 , 8).
Find the coordinate of O , the centre of a circle passing through P (3 , 0), Q (2 , `sqrt 5`) and R (`-2 sqrt 2` , -1). Also find its radius.
P(5 , -8) , Q (2 , -9) and R(2 , 1) are the vertices of a triangle. Find tyhe circumcentre and the circumradius of the triangle.
Point P(0, 2) is the point of intersection of y-axis and perpendicular bisector of line segment joining the points A(–1, 1) and B(3, 3).
The point P(–2, 4) lies on a circle of radius 6 and centre C(3, 5).
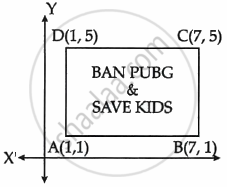
Read the following passage:
|
Use of mobile screen for long hours makes your eye sight weak and give you headaches. Children who are addicted to play "PUBG" can get easily stressed out. To raise social awareness about ill effects of playing PUBG, a school decided to start 'BAN PUBG' campaign, in which students are asked to prepare campaign board in the shape of a rectangle: One such campaign board made by class X student of the school is shown in the figure.
|
Based on the above information, answer the following questions:
- Find the coordinates of the point of intersection of diagonals AC and BD.
- Find the length of the diagonal AC.
-
- Find the area of the campaign Board ABCD.
OR - Find the ratio of the length of side AB to the length of the diagonal AC.
- Find the area of the campaign Board ABCD.