Advertisements
Advertisements
Question
|
In a GPS, The lines that run east-west are known as lines of latitude, and the lines running north-south are known as lines of longitude. The latitude and the longitude of a place are its coordinates and the distance formula is used to find the distance between two places. The distance between two parallel lines is approximately 150 km. A family from Uttar Pradesh planned a round trip from Lucknow (L) to Puri (P) via Bhuj (B) and Nashik (N) as shown in the given figure below.
|
Based on the above information answer the following questions using the coordinate geometry.
- Find the distance between Lucknow (L) to Bhuj (B).
- If Kota (K), internally divide the line segment joining Lucknow (L) to Bhuj (B) into 3 : 2 then find the coordinate of Kota (K).
- Name the type of triangle formed by the places Lucknow (L), Nashik (N) and Puri (P)
[OR]
Find a place (point) on the longitude (y-axis) which is equidistant from the points Lucknow (L) and Puri (P).
Solution
I. LB = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)`
⇒ LB = `sqrt((0 - 5)^2 + (7 - 10)^2)`
LB = `sqrt((5)^2 + (3)^2)`
⇒ LB = `sqrt(25 + 9)`
LB = `sqrt(34)`
Hence the distance is `150 sqrt(34)` km
II. Coordinate of Kota (K) is `((3 xx 5 + 2 xx 0)/(3 + 2), (3 xx 7 + 2 xx 10)/(3 + 2))`
= `((15 + 0)/5, (21 + 20)/5)`
= `(3, 41/5)`
III. L(5, 10), N(2, 6), P(8, 6)
LN = `sqrt((2 - 5)^2 + (6 - 10)^2)`
= `sqrt((3)^2 + (4)^2)`
= `sqrt(9 + 16)`
= `sqrt(25)`
= 5
NP = `sqrt((8 - 2)^2 + (6 - 6)^2)`
= `sqrt((4)^2 + (0)^2)`
= 4
PL = `sqrt((8 - 5)^2 + (6 - 10)^2)`
= `sqrt((3)^2 + (4)^2)`
⇒ LB = `sqrt(9 + 16)`
= `sqrt(25)`
= 5
As LN = PL ≠ NP, So ∆LNP is an isosceles triangle.
[OR]
Let A(0, b) be a point on the y-axis then AL = AP
⇒ `sqrt((5 - 0)^2 + (10 - b)^2) = sqrt((8 - 0)^2 + (6 - b)^2)`
⇒ (5)2 + (10 − b)2 = (8)2 + (6 − b)2
⇒ 25 + 100 − 20b + b2 = 64 + 36 − 12b + b2
⇒ 8b = 25
⇒ b = `25/8`
So, the coordinate on y axis is `(0, 25/8)`
APPEARS IN
RELATED QUESTIONS
Find the values of y for which the distance between the points P (2, -3) and Q (10, y) is 10 units.
Find the distance of the following points from the origin:
(ii) B(-5,5)
Find the distance of the following point from the origin :
(6 , 8)
Find the distance between the following point :
(sin θ , cos θ) and (cos θ , - sin θ)
Find the distance between P and Q if P lies on the y - axis and has an ordinate 5 while Q lies on the x - axis and has an abscissa 12 .
P and Q are two points lying on the x - axis and the y-axis respectively . Find the coordinates of P and Q if the difference between the abscissa of P and the ordinates of Q is 1 and PQ is 5 units.
Find the point on the x-axis equidistant from the points (5,4) and (-2,3).
A circle drawn with origin as the centre passes through `(13/2, 0)`. The point which does not lie in the interior of the circle is ______.
Read the following passage:
|
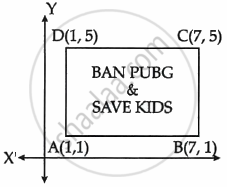
Use of mobile screen for long hours makes your eye sight weak and give you headaches. Children who are addicted to play "PUBG" can get easily stressed out. To raise social awareness about ill effects of playing PUBG, a school decided to start 'BAN PUBG' campaign, in which students are asked to prepare campaign board in the shape of a rectangle: One such campaign board made by class X student of the school is shown in the figure.
|
Based on the above information, answer the following questions:
- Find the coordinates of the point of intersection of diagonals AC and BD.
- Find the length of the diagonal AC.
-
- Find the area of the campaign Board ABCD.
OR - Find the ratio of the length of side AB to the length of the diagonal AC.
- Find the area of the campaign Board ABCD.
|
Tharunya was thrilled to know that the football tournament is fixed with a monthly timeframe from 20th July to 20th August 2023 and for the first time in the FIFA Women’s World Cup’s history, two nations host in 10 venues. Her father felt that the game can be better understood if the position of players is represented as points on a coordinate plane. |
- At an instance, the midfielders and forward formed a parallelogram. Find the position of the central midfielder (D) if the position of other players who formed the parallelogram are :- A(1, 2), B(4, 3) and C(6, 6)
- Check if the Goal keeper G(–3, 5), Sweeper H(3, 1) and Wing-back K(0, 3) fall on a same straight line.
[or]
Check if the Full-back J(5, –3) and centre-back I(–4, 6) are equidistant from forward C(0, 1) and if C is the mid-point of IJ. - If Defensive midfielder A(1, 4), Attacking midfielder B(2, –3) and Striker E(a, b) lie on the same straight line and B is equidistant from A and E, find the position of E.