Advertisements
Advertisements
Question
Read the following passage:
|
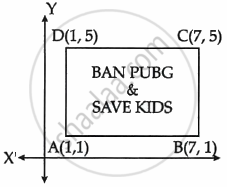
Use of mobile screen for long hours makes your eye sight weak and give you headaches. Children who are addicted to play "PUBG" can get easily stressed out. To raise social awareness about ill effects of playing PUBG, a school decided to start 'BAN PUBG' campaign, in which students are asked to prepare campaign board in the shape of a rectangle: One such campaign board made by class X student of the school is shown in the figure.
|
Based on the above information, answer the following questions:
- Find the coordinates of the point of intersection of diagonals AC and BD.
- Find the length of the diagonal AC.
-
- Find the area of the campaign Board ABCD.
OR - Find the ratio of the length of side AB to the length of the diagonal AC.
- Find the area of the campaign Board ABCD.
Advertisements
Solution
We have, A(1, 1), B(7, 1), (7, 5), D(1, 5)
From these coordinates it is clear that the board is in the shape of rectangle
- Point of intersection of diagonals is their midpoint
So, `[((1 + 7))/2, ((1 + 5))/2]` = (4, 3) - Length of diagonal AC
AC = `sqrt((7 - 1)(7 - 1) + (5 - 1)(5 - 1))= sqrt(52)` units -
- Area of campaign board
= 6 × 4
= 24 units square
OR - Ratio of lengths = `("AB")/("AC")`
= `6/sqrt(52)`
= `6 : sqrt(52)`
- Area of campaign board
APPEARS IN
RELATED QUESTIONS
If the point P(x, y) is equidistant from the points A(a + b, b – a) and B(a – b, a + b). Prove that bx = ay.
If P (2, – 1), Q(3, 4), R(–2, 3) and S(–3, –2) be four points in a plane, show that PQRS is a rhombus but not a square. Find the area of the rhombus
Find the distance between the following point :
(Sin θ - cosec θ , cos θ - cot θ) and (cos θ - cosec θ , -sin θ - cot θ)
Find the coordinates of the points on the y-axis, which are at a distance of 10 units from the point (-8, 4).
A point P lies on the x-axis and another point Q lies on the y-axis.
If the abscissa of point P is -12 and the ordinate of point Q is -16; calculate the length of line segment PQ.
Calculate the distance between A (7, 3) and B on the x-axis whose abscissa is 11.
Find the distance of the following points from origin.
(5, 6)
Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
If a player P needs to be at equal distances from A and G, such that A, P and G are in straight line, then position of P will be given by ______.
A circle has its centre at the origin and a point P(5, 0) lies on it. The point Q(6, 8) lies outside the circle.
Find a point which is equidistant from the points A(–5, 4) and B(–1, 6)? How many such points are there?
