Advertisements
Advertisements
प्रश्न
Consider two ‘postulates’ given below:-
- Given any two distinct points A and B, there exists a third point C which is in between A and B.
- There exist at least three points that are not on the same line.
Do these postulates contain any undefined terms? Are these postulates consistent? Do they follow from Euclid’s postulates? Explain.
उत्तर
Yes, these postulates include undefined terms like 'point and line'. Furthermore, these postulates are consistent because they deal with two distinct situations:
- States that given two points, A and B, a point C exists on the line that connects them. Whereas
- States that given points A and B, you can select a point C that is not on the line that connects them.
No, these postulates are not derived from Euclid's postulates but rather from the axiom, "Given two distinct points, there is a unique line that passes through them."
APPEARS IN
संबंधित प्रश्न
If a point C lies between two points A and B such that AC = BC, then prove that AC = `1/2` AB. Explain by drawing the figure.
Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)
In how many points a line, not in a plane, can intersect the plane?
How many least number of distinct points determine a unique plane?
The total number of propositions in the Elements are ______.
In Indus Valley Civilisation (about 3000 B.C.), the bricks used for construction work were having dimensions in the ratio ______.
Euclid belongs to the country ______.
Solve the following question using appropriate Euclid’s axiom:
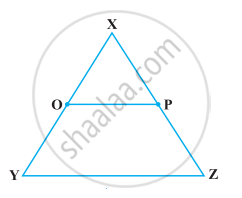
In the following figure, if OX = `1/2` XY, PX = `1/2` XZ and OX = PX, show that XY = XZ.
Read the following statement :
An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third one and all its angles are 60° each.
Define the terms used in this definition which you feel necessary. Are there any undefined terms in this? Can you justify that all sides and all angles are equal in a equilateral triangle.
The following statement is true or false? Give reason for your answer.
In the following figure, if AB = PQ and PQ = XY, then AB = XY.

