Advertisements
Advertisements
प्रश्न
Define mutual inductance between two long coaxial solenoids. Find out the expression for the mutual inductance of inner solenoid of length l having the radius r1 and the number of turns n1 per unit length due to the second outer solenoid of same length and r2 number of turns per unit length.
उत्तर
Mutual Inductance
The ability of production of induced emf in one coil, due to varying current in the neighbouring coil is called mutual inductance.
Magnetic flux, Φ = MI Where, M is called coefficient of mutual induction
Mutual Inductance of Two Long Solenoids
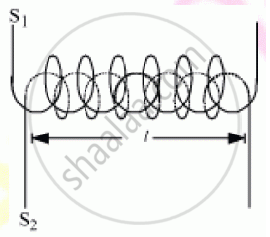
Consider two long solenoids S1 and S2 of same length l, such that solenoid S2 surrounds solenoid S1 completely.
Φ21 = M21I1
Where, M21 is the coefficient of mutual induction of the two solenoids
Magnetic field produced inside solenoid S1 on passing current through it,
B1 = μ0n1I1
Magnetic flux linked with each turn of solenoid S2 will be equal to B1 times the area of cross-section of solenoid S1.
Magnetic flux linked with each turn of the solenoid S2 = B1A
Therefore, total magnetic flux linked with the solenoid S2,
Φ21 = B1A × n2l = μ0n1I1× A× n2l
Φ21 = μ0n1n2lAI1
∴ M21 = μ0n1n2Al
Similarly, the mutual inductance between the two solenoids, when current is passed through solenoid S2 and induced emf is produced in solenoid S1, is given by
M12 = μ0n1n2Al
∴M12 = M21 = M (say)
Hence, coefficient of mutual induction between the two long solenoids
`M = mu_0n_1n_2Al`
संबंधित प्रश्न
Obtain an expression for the energy stored in a solenoid of self-inductance ‘L’ when the current through it grows from zero to ‘I’.
Derive the expression for the magnetic field due to a solenoid of length ‘2l’, radius ‘a’ having ’n’ number of turns per unit length and carrying a steady current ‘I’ at a point
on the axial line, distance ‘r’ from the centre of the solenoid. How does this expression compare with the axial magnetic field due to a bar magnet of magnetic moment ‘m’?
An observer to the left of a solenoid of N turns each of cross section area 'A' observes that a steady current I in it flows in the clockwise direction. Depict the magnetic field lines due to the solenoid specifying its polarity and show that it acts as a bar magnet of magnetic moment m = NIA.
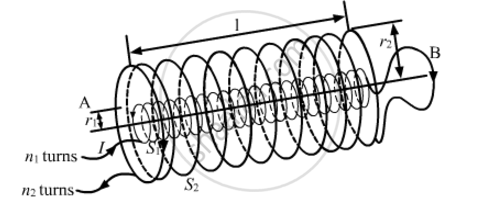
Two long coaxial insulated solenoids, S1 and S2 of equal lengths are wound one over the other as shown in the figure. A steady current "I" flow thought the inner solenoid S1 to the other end B, which is connected to the outer solenoid S2 through which the same current "I" flows in the opposite direction so as to come out at end A. If n1 and n2 are the number of turns per unit length, find the magnitude and direction of the net magnetic field at a point (i) inside on the axis and (ii) outside the combined system
A wire AB is carrying a steady current of 12 A and is lying on the table. Another wire CD carrying 5 A is held directly above AB at a height of 1 mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g = 10 ms−2]
A wire AB is carrying a steady current of 10 A and is lying on the table. Another wire CD carrying 6 A is held directly above AB at a height of 2 mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g = 10 ms−2]
A long solenoid of radius 2 cm has 100 turns/cm and carries a current of 5 A. A coil of radius 1 cm having 100 turns and a total resistance of 20 Ω is placed inside the solenoid coaxially. The coil is connected to a galvanometer. If the current in the solenoid is reversed in direction, find the charge flown through the galvanometer.
A copper wire having resistance 0.01 ohm in each metre is used to wind a 400-turn solenoid of radius 1.0 cm and length 20 cm. Find the emf of a battery which when connected across the solenoid will cause a magnetic field of 1.0 × 10−2 T near the centre of the solenoid.
A tightly-wound, long solenoid carries a current of 2.00 A. An electron is found to execute a uniform circular motion inside the solenoid with a frequency of 1.00 × 108 rev s−1. Find the number of turns per metre in the solenoid.
A tightly-wound, long solenoid is kept with its axis parallel to a large metal sheet carrying a surface current. The surface current through a width dl of the sheet is Kdl and the number of turns per unit length of the solenoid is n. The magnetic field near the centre of the solenoid is found to be zero. (a) Find the current in the solenoid. (b) If the solenoid is rotated to make its axis perpendicular to the metal sheet, what would be the magnitude of the magnetic field near its centre?
