Advertisements
Advertisements
प्रश्न
Derive the law of reflection using Huygen’s Wave Theory.
Advertisements
उत्तर
Huygen’s wave theory :
Reflection at a plane surface : Consider a plane reflecting surface XY. Let AB be a plane wavefront of light incident obliquely on XY. When the incident wavefront touches XY at A, a secondary wavelet starts, spreading from A according to Huygens’ principle. Let the ray at B reach XY at D after a time t. If v is the speed of light in air then BD = vt. During this time t, the secondary wavelet from A spreads over a hemisphere of radius vt. with centre at A. Let CD be a tangent to this hemisphere. Then AC = BD. C and D are in the same phase. If we consider all the points between A and D, then CD will be tangential to all the secondary wavelets originating from these points at the end of t seconds. Hence CD is the reflected wavefront.
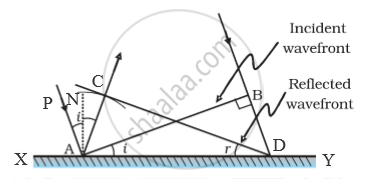
Draw AN normal to XY. Then
`anglePAN = i` , the angle of incidence , and `angleNAC = r` the angle of reflection
In triangles BAD and CDA
AC = BD = vt; AD is common, and `angleABD = angleACD = 90^circ` because the rays are normal to wavefronts.
`therefore` Triangles BAD and CDA are congruent
`therefore angleDAC = angleBDA , 90^circ - r = 90^circ - i`
OR `therefore i = r`
i.e , angle of incidence is equal to angle of reflection .
APPEARS IN
संबंधित प्रश्न
On the basis of Huygens' wave theory of light prove that velocity of light in a rarer medium is greater than velocity of light in a denser medium.
What is the shape of the wavefront in the following case?
Light diverging from a point source.
The refractive indices of water and diamond are `4/3` and 2.42 respectively. Find the speed of light in water and diamond. (c = 3x108 m/s)
Use Huygens’s principle to explain the formation of diffraction pattern due to a single slit illuminated by a monochromatic source of light.
When the width of the slit is made double the original width, how would this affect the size and intensity of the central diffraction band?
Define a wavefront. Using 'Huygens' principle, draw the shape of a refracted wavefront, when a plane wave is incident on a convex lens.
Figure shows a standard two slit arrangement with slits S1, S2, P1, P2 are the two minima points on either side of P (Figure). At P2 on the screen, there is a hole and behind P2 is a second 2-slit arrangement with slits S3, S4 and a second screen behind them.
Is Huygen’s principle valid for longitudinal sound waves?
According to Huygens’s principle, the amplitude of secondary wavelets is ______.
Represent diagrammatically how the incident planar wavefronts of wavelength λ pass through an aperture of size d, when d is approximately equal to λ.
