Advertisements
Advertisements
प्रश्न
Describe geometry of the Young’s double slit experiment with the help of a ray diagram. What is fringe width? Obtain an expression of it. Write the conditions for constructive as well as destructive interference.
उत्तर
i. Let S1 and S2 be the two coherent monochromatic sources that are separated by short distance d. They emit light waves of wavelength λ.
ii. Let D = horizontal distance between screen and source.
iii. Draw S1M and S2N ⊥ AB
OP = perpendicular bisector of the slit.
Since S1P = S2P, the path difference between waves reaching P from S1 and S2 is zero, therefore there is a bright point at P.
iv. Consider a point Q on the screen which is at a distance y from the central point P on the screen. Light waves from S1 and S2 reach at Q simultaneously by covering paths S1Q and S2Q, where they superimpose. 
Derivation:
In Δ S1MQ, (S1Q)2 = (S1M)2 + (MQ)2
`("S"_1"Q")^2 = "D"^2 + ["y" - "d"/2]^2` ....(1)
In `Δ "S"_2"NQ", ("S"_2"Q")^2 = ("S"_2"N")^2 + ("NQ")^2`
∴ `("S"_2"Q")^2 = "D"^2 + ["y" + "d"/2]^2` ....(2)
Subtract equation (1) from (2),
`("S"_2"Q")^2 - ("S"_1"Q")^2 = ["D"^2 + ("y" + "d"/2)^2] - ["D"^2 + ("y" - "d"/2)^2]`
= `"D"^2 + ("y" + "d"/2)^2 - "D"^2 - ("y" - "d"/2)^2`
= `("y" + "d"/2)^2 - ("y" - "d"/2)^2`
= `("y"^2 + "d"^2/4 + "yd") - ("y"^2 + "d"^2/4 - "yd")`
= `"y"^2 + "d"^2/4 + "yd" - "y"^2 - "d"^2/4 + "yd"("S"_2"Q")^2 - ("S"_1"Q")^2`
= 2yd
∴ `("S"_2"Q" + "S"_1"Q")("S"_2"Q" - "S"_1"Q")` = 2yd
∴ `"S"_2"Q" - "S"_1"Q" = (2"yd")/("S"_2"Q" + "S"_1"Q")` ....(3)
If y << D and d << D then, S1Q ≈ S2Q ≈ D
S2Q + S1Q = 2D
∴ Equation (3) becomes,
`"S"_2"Q" - "S"_1"Q" = (2"yd")/(2"D")`
∴ `"S"_2"Q" - "S"_1"Q" = "yd"/"D"`
∴ Δl = `"yd"/"D"` ....(4)
Equation (4) gives the path difference of two interfering light waves. Point Q will be bright if,
Δl = nλ = 2n`lambda/2`
where n = 0, 1, 2,….
∴ `("y"_"n""d")/"D" = "n"λ = 2"n"lambda/2` ........….[From equation (4)]
∴ `"y"_"n" = "n"(lambda"D")/"d"` ….(5)
Equation (5) represents the distance of the nth bright fringe from the central bright fringe.
Point Q will be a dark point if,
`Deltal = (2"n" - 1)lambda/2`
where n = 1, 2, 3,……
∴ `("y"_"n"^'"d")/"D" = (2"n" - 1)lambda/2`
∴ `"y"_"n"^' = (2"n" - 1)(lambda"D")/(2"d") = ("n" - 1/2) (lambda"D")/"d"` .........(6)
Equation (6) represents the distance of nth dark fringe from the central maximum.
Fringe width:
The distance between any two successive dark or any two successive bright fringes is equal. This is called the fringe width and is given by, Fringe width = W = Δy = `"y"_("n" + 1) - "y"_"n" = "y"_"n + 1"^' - "y"_"n"^'`
W = `λ"D"/"d"`
Thus, both dark and bright fringes are equidistant and have equal widths.
Conditions for constructive and destructive interference:
The phase difference between the two waves reaching P, from `"S"_1` and `"S"_2` is given by,
`Deltaphi = "y""d"/"D"((2pi)/lambda)` ..........`(∵ Deltal = "yd"/"D")`
The condition for constructive interference in terms of phase difference is given by,
ΔΦ = n2π, where, n = 0, ±1 ±2
∴ The condition for destructive interference in terms of phase difference is given by
`Deltaphi = ("n" - 1/2)2pi,` where, n = ±1, ±2
APPEARS IN
संबंधित प्रश्न
Write the necessary conditions to obtain sustained interference fringes.
The intensity at the central maximum (O) in a Young’s double slit experimental set-up shown in the figure is IO. If the distance OP equals one-third of the fringe width of the pattern, show that the intensity at point P, would equal `(I_0)/4`.

Answer the following question.
Describe any two characteristic features which distinguish between interference and diffraction phenomena. Derive the expression for the intensity at a point of the interference pattern in Young's double-slit experiment.
What are the two methods for obtaining coherent sources in the laboratory?
Why two light sources must be of equal intensity to obtain a well-defined interference pattern?
What are coherent sources of light?
How do source and images behave as coherent sources?
Discuss the interference in thin films and obtain the equations for constructive and destructive interference for transmitted and reflected light.
A graph is plotted between the fringe-width Z and the distance D between the slit and eye-piece, keeping other adjustment same. The correct graph is
A.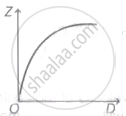 |
B. |
C.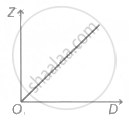 |
D.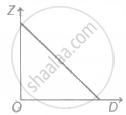 |
The light waves from two independent monochromatic light sources are given by, y1 = 2 sin ωt and y2 = 3 cos ωt. Then the correct statement is ____________.
In Young's experiment for the interference of light, the separation between the silts is d and the distance of the screen from the slits is D. If D is increased by 0.6% and d is decreased by 0.2%, then for the light of a given wavelength, which one of the following is true?
"The fringe width ____________."
In Young's experiment, the distance between the slits is doubled and the distance between the slit and screen is reduced to half, then the fringe width ____________.
In a double slit experiment, the two slits are 2 mm apart and the screen is placed 1 m away. A monochromatic light of wavelength 500 nm is used. What will be the width of each slit for obtaining ten maxima of double slit within the central maxima of single slit pattern?
In a Young's experiment, two coherent sources are placed 0.60 mm apart and the fringes are observed one metre away. If it produces the second dark fringe at a distance of 1 mm from the central fringe, the wavelength of monochromatic light used would be ____________.
Two coherent light sources of intensity ratio 'n' are employed in an interference experiment. The ratio of the intensities of the maxima and minima in the interference pattern is (I1 > I2).
If two waves represented by `"y"_1 = 3 "sin" omega "t"` and `"y"_2 = 5 "sin" (omega "t" + pi/3)` interfere at a point, then the amplitude of the resulting wave will be about ____________.
Two identical light sources s1 and s2 emit light of same wavelength `lambda`. These light rays will exhibit interference if their ______.
Light waves from two coherent sources arrive at two points on a screen with a path difference of zero and λ/2. The ratio of the intensities at the points is ______
A double slit experiment is immersed in water of refractive index 1.33. The slit separation is 1 mm, distance between slit and screen is 1.33 m. The slits are illuminated by a light of wavelength 6300 Å. The fringe width is ______.
In Young's double-slit experiment, the distance between the slits is 3 mm and the slits are 2 m away from the screen. Two interference patterns can be obtained on the screen due to light of wavelength 480 nm and 600 run respectively. The separation on the screen between the 5th order bright fringes on the two interference patterns is ______
What is meant by Constructive interference?
White light is passed through a double slit and interference is observed on a screen 1.5 m away. The separation between the slits is 0.3 mm. The first violet and red fringes are formed 2.0 mm and 3.5 mm away from the central white fringes. The difference in wavelengths of red and violet light is ______ nm.
Two coherent sources P and Q produce interference at point A on the screen where there is a dark band which is formed between 4th bright band and 5th bright band. Wavelength of light used is 6000 Å. The path difference between PA and QA is ______.
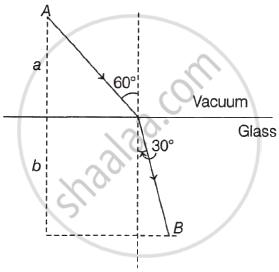
A ray of light AO in vacuum is incident on a glass slab at angle 60° and refracted at angle 30° along OB as shown in the figure. The optical path length of light ray from A to B is ______.
Describe Young's double-slit interference experiment.
