Advertisements
Advertisements
प्रश्न
Draw the graph of the following linear equation in two variable : `(x-2)/3 = y - 3`
उत्तर
We have
`(x-2)/3 = y - 3`
⇒ ` x - 2 = 3 (y -3) `
⇒ ` x - 2 = 3y - 9 `
⇒ ` x = 3y - 9 + 2`
⇒ `x = 3y - 7`
Putting y = 0 , we get x - 0 = -7 ⇒ x = - 7
Putting y = 2 , we get x - 3 (2) = - 7 ⇒ x =-1
Putting y = 3 , we get x = 3 ( 3) - 7 ⇒ x = 2
Thus, we get the following table giving the two points on the line represented by the
equation `(x -2)/y ` = y - 3
Graph for the equation `(x -2)/y ` = y - 3

APPEARS IN
संबंधित प्रश्न
If the point (3, 4) lies on the graph of the equation 3y = ax + 7, find the value of a.
The taxi fare in a city is as follows:- For the first kilometre, the fare is Rs. 8 and for the subsequent distance it is Rs. 5 per km. Taking the distance covered as x km and total fare as Rs y, write a linear equation for this information, and draw its graph.
Draw the graph of the following linear equations in two variable : 2𝑦 = −𝑥 + 1
Plot the points (3, 5) and (− 1, 3) on a graph paper and verify that the straight line passing
through these points also passes through the point (1, 4).
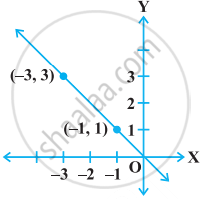
From the choices given below, choose the equation whose graph is given in Fig. below.
(i) y = x (ii) x + y = 0 (iii) y = 2x (iv) 2 + 3y = 7x

[Hint: Clearly, (-1, 1) and (1, -1) satisfy the equation x + y = 0]
If the point (2, -2) lies on the graph of the linear equation 5x + ky = 4, find the value of k.
Draw the graph of the equation
4x + 3y + 6 = 0
From the graph, find :
(i) y1, the value of y, when x = 12.
(ii) y2, the value of y, when x = - 6.
By drawing a graph for each of the equations 3x + y + 5 = 0; 3y - x = 5 and 2x + 5y = 1 on the same graph paper; show that the lines given by these equations are concurrent (i.e. they pass through the same point). Take 2 cm = 1 unit on both the axes.
Draw the graph for each of the following equation: Also, find the coordinates of the points where the graph of the equation meets the coordinate axes:
`(1)/(2) x + (1)/(3) y` = 1
The graph given below represents the linear equation x + y = 0.