Advertisements
Advertisements
प्रश्न
Evaluate the following limits:
`lim_(x -> oo) ((x^2 - 2x + 1)/(x^2 -4x + 2))^x`
उत्तर
`lim_(x -> oo) ((x^2 - 2x + 1)/(x^2 -4x + 2))^x = lim_(x -> oo) ((x^2 - 4x + 2 + 2x - 1)/(x^2 - 4x + 2))^x`
= `lim_(x -> oo) [(x^2 - 4x - 2)/(x^2 - 4x + 2) +(2x - 1)/(x^2 - 4x + 2)]^x`
= `lim_(x -> oo) [1 + (2x - 1)/(x^2 - 4x + 2)]^x`
= `lim_(x - oo) [1 + 1/((x^2 -4x + 2)/(2x - 1))]^(((x^2 - 4x + 2)/(2x - 1) xx ((2x - 1)x)/(x^2 - 4x + 2))`
= `lim_(x -> oo) [(1 + (2x - 1)/(x^2 - 4x + 2))^((x^2 - 4x + 2)/(2x - 1))]^(((2x - 1)x)/(x^2 - 4x + 2))`
`lim_(x -> oo) ((x^2 - 2x + 1)/(x^2 - 4x + 2))^x = [lim_(x -> oo) "e"]^((2x^2 - x)/(x^2 - 4x + 2))`
`lim_(x -> oo) (1 + 1/x)^x` = e
= `"e"^(lim_(x ->oo)) ((2x^2 - x)/(x^2 - 4x + 2))`
= `"e"^(lim_(x -> oo) (x^2(2 -x/x^2))/(x^2(1 - (4x)/(x^2) + 2/x^2))`
= `"e"^(lim_(x ->oo) ((2 - 1/x)/(1 -4/x + 2/x^2))`
= `"e"^(((2 - 0)/(1 - 0 + 0))`
`lim_(x -> oo) ((x^2 - 2x + 1)/(x^2 -4x + 2))^x` = e2
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit :
`lim_(y -> 1)[(2y - 2)/(root(3)(7 + y) - 2)]`
Evaluate the following :
Given that 7x ≤ f(x) ≤ 3x2 – 6 for all x. Determine the value of `lim_(x -> 3) "f"(x)`
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 3) (4 - x)`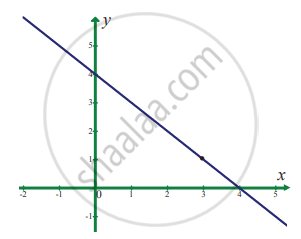
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 5) |x - 5|/(x - 5)`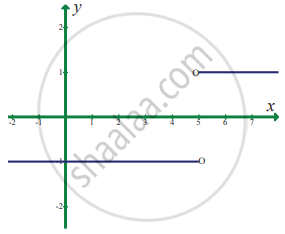
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 0) sec x`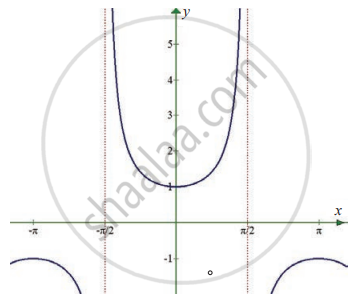
If f(2) = 4, can you conclude anything about the limit of f(x) as x approaches 2?
Evaluate : `lim_(x -> 3) (x^2 - 9)/(x - 3)` if it exists by finding `f(3^-)` and `f(3^+)`
Evaluate the following limits:
`lim_(sqrt(x) -> 3) (x^2 - 81)/(sqrt(x) - 3)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(1 - x) - 1)/x^2`
Evaluate the following limits:
`lim_(x -> oo) (x^4 - 5x)/(x^2 - 3x + 1)`
Show that `lim_("n" -> oo) (1^2 + 2^2 + ... + (3"n")^2)/((1 + 2 + ... + 5"n")(2"n" + 3)) = 9/25`
Evaluate the following limits:
`lim_(x -> oo)(1 + "k"/x)^("m"/x)`
Evaluate the following limits:
`lim_(x -> oo) ((2x^2 + 3)/(2x^2 + 5))^(8x^2 + 3)`
Evaluate the following limits:
`lim_(x -> oo) (1 + 3/x)^(x + 2)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(1 + sinx) - sqrt(1 - sinx))/tanx`
Choose the correct alternative:
`lim_(x - pi/2) (2x - pi)/cos x`
Choose the correct alternative:
`lim_(x -> oo) ((x^2 + 5x + 3)/(x^2 + x + 3))^x` is
Choose the correct alternative:
If `lim_(x -> 0) (sin "p"x)/(tan 3x)` = 4, then the value of p is
Choose the correct alternative:
`lim_(x -> oo) (1/"n"^2 + 2/"n"^2 + 3/"n"^2 + ... + "n"/"n"^2)` is
