Advertisements
Advertisements
प्रश्न
Evaluate the following limits:
`lim_(x -> 0) ("e"^x - "e"^(-x))/sinx`
उत्तर
We know `lim_(x -> 0) ("e"^x - 1)/x` = 1
`lim_(x -> 0) sinx/x` = 1
`lim_(x -> 0) ("e"^x - "e"^(-x))/sinx = lim_(x -> 0) ("e"^x - 1/"e"^x)/sinx`
= `lim_(x -> 0) (("e"^x * "e"^x - 1)/"e"^x)/(sinx)`
= `lim_(x -> 0) ("e"^(2x) - 1)/("e"^x sinx)`
= `lim_(x -> 0) (1/"e"^x xx ("e"^(2x) - 1)/(1/2 xx 2x) xx x/sinx)`
= `(lim_(x -> 0) 1/"e"^x) 2(lim_(2x -> 0) ("e"^(2x) - 1)/(2x)) xx 1/((lim_(x -> 0) sinx/x))`
= `1/"e"^0 xx 2 xx 1 xx 1/1`
= `1/1 xx 2 xx 1`
`lim_(x -> 0) ("e"^x - "e"^(-x))/sinx` = 2
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
`lim_(x -> 2)[(x^(-3) - 2^(-3))/(x - 2)]`
Evaluate the following limit :
If `lim_(x -> 5) [(x^"k" - 5^"k")/(x - 5)]` = 500, find all possible values of k.
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) (x^2 + 2)`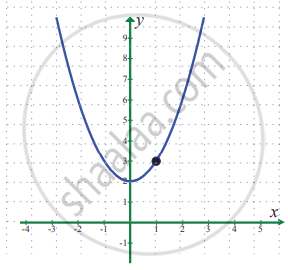
Evaluate : `lim_(x -> 3) (x^2 - 9)/(x - 3)` if it exists by finding `f(3^-)` and `f(3^+)`
Evaluate the following limits:
`lim_("h" -> 0) (sqrt(x + "h") - sqrt(x))/"h", x > 0`
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x + 4) - 3)/(x - 5)`
Evaluate the following limits:
`lim_(x -> 0) (sqrt(1 + x) - 1)/x`
Evaluate the following limits:
`lim_(x -> 3) (x^2 - 9)/(x^2(x^2 - 6x + 9))`
Evaluate the following limits:
`lim_(x -> oo) (x^4 - 5x)/(x^2 - 3x + 1)`
Evaluate the following limits:
`lim_(x -> oo)(1 + 1/x)^(7x)`
Evaluate the following limits:
`lim_(x -> 0)(1 + x)^(1/(3x))`
Evaluate the following limits:
`lim_(x -> pi) (1 + sinx)^(2"cosec"x)`
Evaluate the following limits:
`lim_(x -> ) (sinx(1 - cosx))/x^3`
Choose the correct alternative:
`lim_(x -> oo) ((x^2 + 5x + 3)/(x^2 + x + 3))^x` is
Choose the correct alternative:
`lim_(x -> 3) [x]` =
Choose the correct alternative:
`lim_(alpha - pi/4) (sin alpha - cos alpha)/(alpha - pi/4)` is
Choose the correct alternative:
`lim_(x -> 0) ("e"^(sin x) - 1)/x` =
`lim_(x→-1) (x^3 - 2x - 1)/(x^5 - 2x - 1)` = ______.
The value of `lim_(x→0)(sin(ℓn e^x))^2/((e^(tan^2x) - 1))` is ______.
