Advertisements
Advertisements
प्रश्न
Examine the continuity of the function
\[f\left( x \right) = \left\{ \begin{array}{l}3x - 2, & x \leq 0 \\ x + 1 , & x > 0\end{array}at x = 0 \right.\]
Also sketch the graph of this function.
उत्तर
Meaning of continuity of function - If we talk about a general meaning of continuity of a function f(x) , we can say that if we plot the coordinates (x, f(x)) and try to join all those points in the specified region, we can do so without picking our pen i.e you will put your pen/pencil on graph paper and you can draw the curve without any breakage.
Mathematically we define the same thing as given below:
A function f(x) is said to be continuous at x = c where c is x-coordinate of the point at which continuity is to be checked
If:
`lim_(h->0)f(c - h) = lim_(h->0)f(c + h) = f(c)`
where h is a very small positive no (can assume h = 0.00000000001 like this)
It means :-
Limiting value of the left neighbourhood of x = c also called left hand limit LHL `{i.e lim_(h->0)f(c - h)}` must be equal to limiting value of right neighbourhood of x= c called right hand limit RHL `{i.e lim_(h->0)f(c + h)}` and both must be equal to the value of f(x) at x = c i.e. f(c).
Thus, it is the necessary condition for a function to be continuous.
So, whenever we check continuity we try to check above equality if it holds true, function is continuous else it is discontinuous.
Lets solve now:
Given function is
f(x) = `{(3x - 2, if x ≤ 0),(x + 1, if x > 0):}` ...(2)
We need to check whether f(x) is continuous at x=0 or not
For this we need to check LHL, RHL and value of function at x = 0
Clearly,
f(0) = 3*0 - 2 = -2 [from equation 2]
LHL = `lim_(h->0)f(0 - h) = lim_(h->0)f(-h) = lim_(h->0){3(-h)-2} = -2`
RHL = `lim_(h->0)f(0 + h) = lim_(h->0)f(h) = lim_(h->0){h + 1} = 0 + 1 = 1`
As, LHL ≠ RHL
f(x) is discontinuous at x = 0
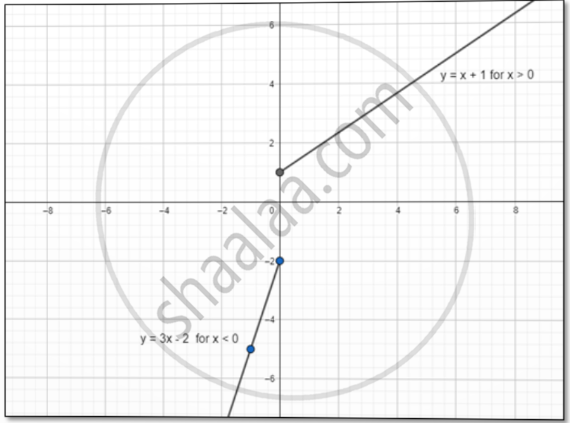
This can also be proved by plotting f(x) on cartesian plane.
For x >0, we need to plot
y = x + 1
put y = 0, we get x = -1 and for second point we put x = 0 and thus get y=1
two points are enough to plot the straight line.
Two coordinates are (-1,0) and (0,1)
For x ≤ 0, we need to plot
y = 3x - 2
put x = 0 then y = -2
on putting y = 0 we get x 2/3
two coordinates are (0, -2) and `(2/3, 0)`
Graph:
APPEARS IN
संबंधित प्रश्न
If f (x) is continuous on [–4, 2] defined as
f (x) = 6b – 3ax, for -4 ≤ x < –2
= 4x + 1, for –2 ≤ x ≤ 2
Show that a + b =`-7/6`
Is the function defined by `f(x) = x^2 - sin x + 5` continuous at x = π?
Find the values of k so that the function f is continuous at the indicated point.
`f(x) = {(kx + 1, "," if x <= 5),(3x - 5, "," if x > 5):} " at x " = 5`
Show that the function defined by f(x) = |cos x| is a continuous function.
Find the values of a so that the function
Find the values of a and b so that the function f given by \[f\left( x \right) = \begin{cases}1 , & \text{ if } x \leq 3 \\ ax + b , & \text{ if } 3 < x < 5 \\ 7 , & \text{ if } x \geq 5\end{cases}\] is continuous at x = 3 and x = 5.
Discuss the continuity of the function
Find the points of discontinuity, if any, of the following functions:
Find the points of discontinuity, if any, of the following functions: \[f\left( x \right) = \begin{cases}\frac{\sin x}{x} + \cos x, & \text{ if } x \neq 0 \\ 5 , & \text { if } x = 0\end{cases}\]
In the following, determine the value of constant involved in the definition so that the given function is continuou: \[f\left( x \right) = \begin{cases}\frac{\sin 2x}{5x}, & \text{ if } x \neq 0 \\ 3k , & \text{ if } x = 0\end{cases}\]
In the following, determine the value of constant involved in the definition so that the given function is continuou: \[f\left( x \right) = \begin{cases}2 , & \text{ if } x \leq 3 \\ ax + b, & \text{ if } 3 < x < 5 \\ 9 , & \text{ if } x \geq 5\end{cases}\]
If \[f\left( x \right) = \frac{\tan\left( \frac{\pi}{4} - x \right)}{\cot 2x}\]
for x ≠ π/4, find the value which can be assigned to f(x) at x = π/4 so that the function f(x) becomes continuous every where in [0, π/2].
Discuss the continuity of f(x) = sin | x |.
Show that the function g (x) = x − [x] is discontinuous at all integral points. Here [x] denotes the greatest integer function.
If \[f\left( x \right) = \begin{cases}\frac{x}{\sin 3x}, & x \neq 0 \\ k , & x = 0\end{cases}\] is continuous at x = 0, then write the value of k.
Determine whether \[f\left( x \right) = \binom{\frac{\sin x^2}{x}, x \neq 0}{0, x = 0}\] is continuous at x = 0 or not.
If \[f\left( x \right) = \begin{cases}\frac{\log\left( 1 + ax \right) - \log\left( 1 - bx \right)}{x}, & x \neq 0 \\ k , & x = 0\end{cases}\] and f (x) is continuous at x = 0, then the value of k is
If the function f (x) defined by \[f\left( x \right) = \begin{cases}\frac{\log \left( 1 + 3x \right) - \log \left( 1 - 2x \right)}{x}, & x \neq 0 \\ k , & x = 0\end{cases}\] is continuous at x = 0, then k =
Find the values of a and b so that the function
If \[f \left( x \right) = \sqrt{x^2 + 9}\] , write the value of
If \[f\left( x \right) = \begin{cases}\frac{\left| x + 2 \right|}{\tan^{- 1} \left( x + 2 \right)} & , x \neq - 2 \\ 2 & , x = - 2\end{cases}\] then f (x) is
If \[f\left( x \right) = a\left| \sin x \right| + b e^\left| x \right| + c \left| x \right|^3\]
If \[f\left( x \right) = \begin{cases}\frac{1 - \cos x}{x \sin x}, & x \neq 0 \\ \frac{1}{2} , & x = 0\end{cases}\]
then at x = 0, f (x) is
Let f(x) = |sin x|. Then ______.
`lim_("x" -> 0) ("x cos x" - "log" (1 + "x"))/"x"^2` is equal to ____________.
The value of f(0) for the function `f(x) = 1/x[log(1 + x) - log(1 - x)]` to be continuous at x = 0 should be
The function f(x) = x2 – sin x + 5 is continuous at x =
What is the values of' 'k' so that the function 'f' is continuous at the indicated point
Find the values of `a` and ` b` such that the function by:
`f(x) = {{:(5",", if x ≤ 2),(ax + b",", if 2 < x < 10),(21",", if x ≥ 10):}`
is a continuous function.
The value of ‘k’ for which the function f(x) = `{{:((1 - cos4x)/(8x^2)",", if x ≠ 0),(k",", if x = 0):}` is continuous at x = 0 is ______.
The function f(x) = x |x| is ______.
