Advertisements
Advertisements
प्रश्न
Examine the continuity of the following:
|x + 2| + |x – 1|
उत्तर
Let f(x) = |x + 2| + |x – 1|
f(x) is defined for all points of R.
Let x0 be an arbitrary point in R.
Then `lim_(x -> x_0)f(x) = lim_(x -> x_0) (|x + 2| + |x - 1|)`
= `|x_0 + 2| + |x_0 - 1|` .......(1)
`f(x_0) = |x_0+ 2| + |x_0 - 1|` .......(2)
From equation (1) and (2) we get
`lim_(x -> x_0)f(x) = f(x_0)`
Thus the limit of the function f(x) exist at x = x0 and is equal to the value of the function at x = x0.
Since x = x0 is an arbitrary point in R, the above
result is true for all points in R.
Hence f(x) is continuous at all points of R.
APPEARS IN
संबंधित प्रश्न
Examine the continuity of the following:
x + sin x
Examine the continuity of the following:
x2 cos x
Examine the continuity of the following:
ex tan x
Examine the continuity of the following:
x . log x
Examine the continuity of the following:
`sinx/x^2`
Examine the continuity of the following:
`(x^2 - 16)/(x + 4)`
Examine the continuity of the following:
`|x - 2|/|x + 1|`
Examine the continuity of the following:
cot x + tan x
At the given point x0 discover whether the given function is continuous or discontinuous citing the reasons for your answer:
x0 = 3, `f(x) = {{:((x^2 - 9)/(x - 3)",", "if" x ≠ 3),(5",", "if" x = 3):}`
For what value of `alpha` is this function `f(x) = {{:((x^4 - 1)/(x - 1)",", "if" x ≠ 1),(alpha",", "if" x = 1):}` continuous at x = 1?
If f and g are continuous functions with f(3) = 5 and `lim_(x -> 3) [2f(x) - g(x)]` = 4, find g(3)
The function `f(x) = (x^2 - 1)/(x^3 - 1)` is not defined at x = 1. What value must we give f(1) inorder to make f(x) continuous at x =1?
State how continuity is destroyed at x = x0 for the following graphs.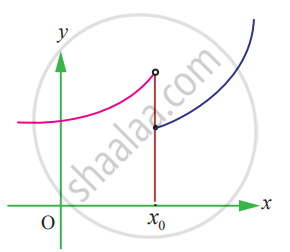
Choose the correct alternative:
If f : R → R is defined by `f(x) = [x - 3] + |x - 4|` for x ∈ R then `lim_(x -> 3^-) f(x)` is equal to
Choose the correct alternative:
The value of `lim_(x -> "k") x - [x]`, where k is an integer is
Choose the correct alternative:
Let a function f be defined by `f(x) = (x - |x|)/x` for x ≠ 0 and f(0) = 2. Then f is
