Advertisements
Advertisements
प्रश्न
Factorise the following:
2a2 + 9a + 10
उत्तर
Product = 2 × 10 = 20, sum = 9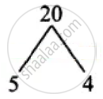
Split the middle term as 5a and 4a
2a2 + 9a + 10 = 2a2 + 5a + 4a + 10
= a(2a + 5) + 2(2a + 5)
= (2a+ 5)(a+ 2)
APPEARS IN
संबंधित प्रश्न
Identify polynomials in the following:
`q(x)=2x^2-3x+4/x+2`
If `f(x) = 2x^2 - 13x^2 + 17x + 12` find f(2)
If the polynomials 2x3 + ax2 + 3x − 5 and x3 + x2 − 4x +a leave the same remainder when divided by x −2, find the value of a.
f(x) = x5 + 3x4 − x3 − 3x2 + 5x + 15, g(x) = x + 3
For what value of a is (x − 5) a factor of x3 − 3x2 + ax − 10?
If x − 2 is a factor of the following two polynomials, find the values of a in each case x5 − 3x4 − ax3 + 3ax2 + 2ax + 4.
In the following two polynomials, find the value of a, if x − a is factor x6 − ax5 + x4 − ax3 + 3x − a + 2.
x4 + 10x3 + 35x2 + 50x + 24
If (3x − 1)7 = a7x7 + a6x6 + a5x5 +...+ a1x + a0, then a7 + a5 + ...+a1 + a0 =
If both x − 2 and \[x - \frac{1}{2}\] are factors of px2 + 5x + r, then
