Advertisements
Advertisements
प्रश्न
\[\ce{Fe+}\] ions are accelerated through a potential difference of 500 V and are injected normally into a homogeneous magnetic field B of strength 20.0 mT. Find the radius of the circular paths followed by the isotopes with mass numbers 57 and 58. Take the mass of an ion = A (1.6 × 10−27) kg, where A is the mass number.
उत्तर
Given:
Potential difference through which the Fe+ ions are accelerated, V = 500 V
Strength of the homogeneous magnetic field, B = 20.0 mT = 20 × 10−3 T
Mass numbers of the two isotopes are 57 and 58.
Mass of an ion = A (1.6 × 10−27) kg
We know that the radius of the circular path described by a particle in a magnetic field,
`r = (mv)/(qB)`
For isotope 1,
`r_1= (m_1v_1)/(qB)`
For isotope 2,
`r_2 = (m_2v_2)/(qB)`
⇒`(r_1)/(r_2)=(m_2v_1)/(m_2v_2)`
As both the isotopes are accelerated via the same potential V, the K.E gained by the two particles will be same.
`qV = 1/2 m_1v_1^2 = 1/2m_2v_2^2`
`(m_1)/(m_2) = (v_1^2)/(v_2^2)`
⇒ `(r_1)/(r_2) = ((m_1)/(m_2))^{3/2}`
Also, r1 = `(mv_1)/(qB)`
= `(m_1 sqrt(2qv)/m_1)/(qB)`
= `1/B sqrt ((2m_1V)/q)`
= `sqrt(1000xx57xx1.6xx10^-27)/sqrt (1.6 xx 10^-19 xx 20xx10^-3)`
= `1.19 xx 10^-2m = 119 cm`
For the second isotope:
`As (r_1)/r_2 = ((m_1)/(m_2))^{3/2}`
`r_2 = ((m_2)/(m_1))^{3/2} r_1`
= `(58/57)3/2 xx 119 cm`
= 120 cm
APPEARS IN
संबंधित प्रश्न
In a cyclotron, magnetic field of 3·5Wb/m2 is used to accelerate protons. What should be the time interval in which the electric field between the Dees be reversed?
(Mass of proton = 1· 67 x 10-27Kg, Charge on proton =1·6x10-19c).
State the principle of a cyclotron.
Show that the time period of revolution of particles in a cyclotron is independent of their speeds. Why is this property necessary for the operation of a cyclotron?
Deduce an expression for the frequency of revolution of a charged particle in a magnetic field and show that it is independent of velocity or energy of the particle.
A deuteron and a proton are accelerated by the cyclotron. Can both be accelerated with the same oscillator frequency? Give reason to justify your answer.
Explain the principle and working of a cyclotron with the help of a schematic diagram. Write the expression for cyclotron frequency.
Verify that the units weber and volt second are the same.
Which of the following particles will describe the smallest circle when projected with the same velocity perpendicular to a magnetic field?
If a charged particle kept at rest experiences an electromagnetic force,
(a) there must be an electric field
(b) there must be a magnetic field
(c) both fields cannot be zero
(d) both fields can be non-zero
An electron is projected horizontally with a kinetic energy of 10 keV. A magnetic field of strength 1.0 × 10−7 T exists in the vertically upward direction.
(a) Will the electron deflect towards the right or left of its motion?
(b) Calculate the sideways deflection of the electron while travelling through 1 m. Make appropriate approximations.
Consider a 10-cm long portion of a straight wire carrying a current of 10 A placed in a magnetic field of 0.1 T making an angle of 53° with the wire. What magnetic force does the wire experience?
Figure shows a rod PQ of length 20.0 cm and mass 200 g suspended through a fixed point O by two threads of lengths 20.0 cm each. A magnetic field of strength 0.500 T exists in the vicinity of the wire PQ, as shown in the figure. The wires connecting PQ with the battery are loose and exert no force on PQ. (a) Find the tension in the threads when the switch S is open. (b) A current of 2.0 A is established when the switch S is closed. Find the tension in the threads now.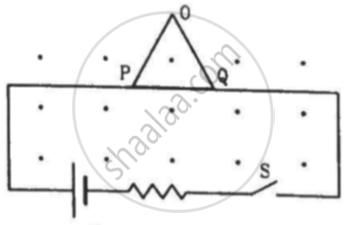
A cyclotron's oscillator frequency is 10 MHz. What should be the operating magnetic field for accelerating protons? If the radius of its 'dees' is 60 cm, calculate the kinetic energy (in MeV) of the proton beam produced by the accelerator.
The cyclotron was designed by ______
A cyclotron can accelerate ______.
In a cyclotron, a charged particle ______.
Describe the motion of a charged particle in a cyclotron if the frequency of the radio frequency (rf) field were doubled.
