Advertisements
Advertisements
प्रश्न
Find the area of the circle in which a square of area 64 cm2 is inscribed. [Use π = 3.14]
उत्तर
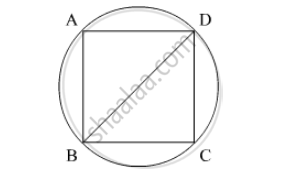
We have given area of the square.
`∴ "side"^2=64`
`∴ "side"=8`
Now we will find the diameter of the square.
`∴ "diagonal"=sqrt3xx"side"`
`∴ "diagonal"=sqrt2xx8`
`∴"diagonal"=8sqrt2`
We know that diagonal of the square is same as the diameter of the circle.
`∴ "diameter"=8sqrt2`
`∴ "radius"=4sqrt2`
Now we will find the area of the circle as shown below.
`∴ "area opf the circle"=pi xxr^2`
`∴ "area of the circle"= pixx4sqrt2xx4sqrt2`
`∴ "area of the circle"=3.14xx16xx2`
`∴ "area of the circle"=3.14xx32`
`∴ "area of the circle"=100.48`
`"Therefore, area of the circle is" 100.48 cm^2`
APPEARS IN
संबंधित प्रश्न
Find the perimeter of the adjoining figure, which is a semicircle including its diameter.
An archery target has three regions formed by three concentric circles as shown in figure. If the diameters of the concentric circles are in the ratios 1 : 2 : 3, then find the ratio of the areas of three regions.
The area of sector is one-twelfth that of the complete circle. Find the angle of the sector .
In the following figure, ABCD is a rectangle with AB = 14 cm and BC = 7 cm. Taking DC, BC and AD as diameters, three semi-circles are drawn as shown in the figure. Find the area of the shaded region.
If a chord of a circle of radius 28 cm makes an angle of 90 ° at the centre, then the area of the major segment is
If the area of a circle is equal to the sum of the areas of two circles of diameters 10 cm and 24 cm, then diameter of the large circle (in cm) is
In the given figure, O is the centre of the bigger circle, and AC is its diameter. Another circle with AB as diameter is drawn. If AC = 54 cm and BC = 10, find the area of the shaded region.
In the given figure, an equilateral triangle has been inscribed in a circle of radius 4 cm. Find the area of the shaded region.

The radii of two circles are in the ratio 5 : 8. If the difference between their areas is 156p cm2, find the area of the bigger circle.
What is the diameter of a circle whose area is equal to the sum of the areas of two circles of radii 40cm and 9cm?
