Advertisements
Advertisements
प्रश्न
If a chord of a circle of radius 28 cm makes an angle of 90 ° at the centre, then the area of the major segment is
पर्याय
392 cm2
1456 cm2
1848 cm2
2240 cm2
उत्तर
Area of major segment,
`="Area of circle"=[piθ/360-sin θ/2 cos θ/2](r^2)^2`
`= pi(28)^2-(pi/4-1/2)(28)^2`
`=784pi-196(pi-2)`
`=2240 cm^2`
APPEARS IN
संबंधित प्रश्न
Find the area and perimeter of an isosceles right angled triangle, each of whose equal sides measure 10cm.
In the following figure, PQRS is a square of side 4 cm. Find the area of the shaded square.
In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

The perimeter of a triangle is 30 cm and the circumference of its incircle is 88 cm. The area of the triangle is
The area of a sector whose perimeter is four times its radius r units, is
If the area of a circle is equal to the sum of the areas of two circles of diameters 10 cm and 24 cm, then diameter of the large circle (in cm) is
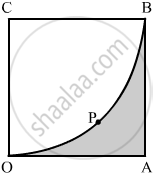
In the given figure, OABC is a square of side 7 cm. If COPB is a quadrant of a circle with centre C find the area of the shaded region.
The length of an arc of a circle, subtending an angle of 54° at the centre, is 16.5 cm. Calculate the radius, circumference and area of the circle.
Two circles touch externally. The sum of their areas is 130π sq. cm and the distance between their centers is 14 cm. Find the radii of the circles.
The diameters of three wheels are in the ratio 2 : 4 : 8. If the sum of the circumferences of these circles be 132 cm, find the difference between the areas of the largest and the smallest of these wheels.
