Advertisements
Advertisements
प्रश्न
Find the equation of a line whose slope and y-intercept are m = `2/3`, c = -2
उत्तर
m = `2/3`, c = -2
y = mx + c
y = `2/3`x -2
⇒ -2x + 3y + 6 = 0
2x - 3y - 6 = 0
⇒ 2x - 3y = 6
APPEARS IN
संबंधित प्रश्न
A(2, 5), B(–1, 2) and C(5, 8) are the vertices of a triangle ABC, `M' is a point on AB such that AM: MB = 1: 2. Find the coordinates of 'M'. Hence find the equation of the line passing through the points C and M
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(–5, 0)
The line given by the equation `2x - y/3 = 7` passes through the point (k, 6); calculate the value of k.
The line y = 3x – 2 bisects the join of (a, 3) and (2, −5), find the value of a.
A straight line passes through the point (3, 2) and the portion of this line, intercepted between the positive axes, is bisected at this point. Find the equation of the line.
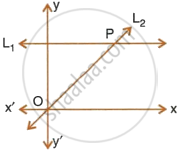
Given equation of line L1 is y = 4.
- Write the slope of line L2 if L2 is the bisector of angle O.
- Write the co-ordinates of point P.
- Find the equation of L2.
Find the value of a line parallel to the following line:
`(2"x")/5 + "y"/3` = 2
Find the equation of a line passing through the intersection of x + 2y + 1= 0 and 2x - 3y = 12 and perpendicular to the line 2x + 3y = 9
A line AB meets X-axis at A and Y-axis at B. P(4, –1) divides AB in the ration 1 : 2.
- Find the co-ordinates of A and B.
- Find the equation of the line through P and perpendicular to AB.

Find the equations of a line passing through the point (2, 3) and having the x – interecpt of 4 units.
